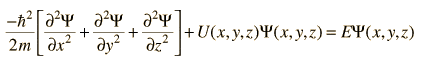- #1
moriheru
- 273
- 17
This is a rather naive question concerning the dimension of the schrodinger equation. If the Schrodinger equation can be wrtiten in a three dimensional form using the laplacian operator can it be written in a 4d version. I understand that the schrodinger equation shows the development of the state of a particle in time... as I said naive!