- #1
Happiness
- 695
- 31
How do we get (6.265)?
Shouldn't we have
##exp(-i\frac{\alpha}{2}\hat{n}.\sigma)=\cos(\frac{\alpha}{2}\hat{n}.\sigma)-i\sin(\frac{\alpha}{2}\hat{n}.\sigma)##?

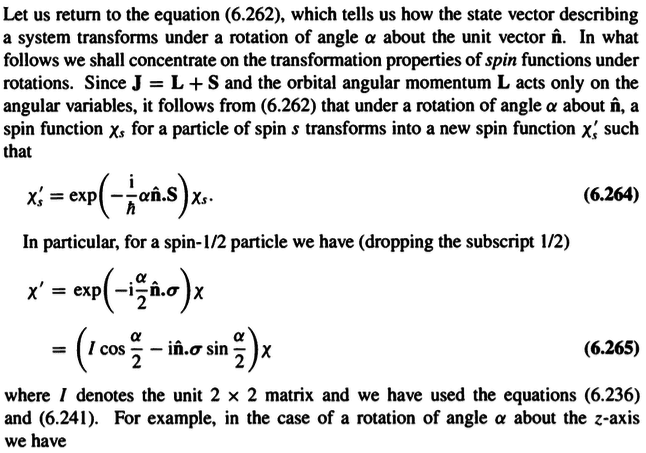


Shouldn't we have
##exp(-i\frac{\alpha}{2}\hat{n}.\sigma)=\cos(\frac{\alpha}{2}\hat{n}.\sigma)-i\sin(\frac{\alpha}{2}\hat{n}.\sigma)##?