PainterGuy
- 938
- 73
Hi,
Originally, the absolute temperature was thought to be around -273 Celsius around 1750 and it was the result extrapolation of of ideal gas law as shown below. I find it hard to phrase my question. But the question is how come they were so confident that the relationship between the volume or pressure for an ideal gas law is going be stay linear until the very limit of absolute zero? One example comes to my mind of Hooke's Law. It is linear only to a certain limit therefore you just can't extrapolate the data. Could you please help me with it?
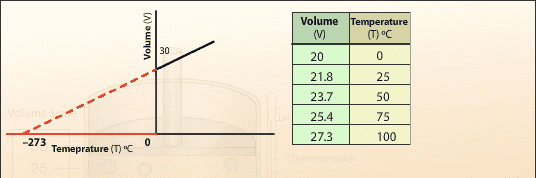
Source: http://www.passmyexams.co.uk/GCSE/physics/volume-temperature-relationship-of-gas-Charles-law.htmlHelpful links:
1: https://en.wikipedia.org/wiki/Absolute_zero#History
2: http://chemed.chem.purdue.edu/genchem/topicreview/bp/ch4/gaslaws3.html
3: https://en.wikipedia.org/wiki/Timeline_of_low-temperature_technology
Originally, the absolute temperature was thought to be around -273 Celsius around 1750 and it was the result extrapolation of of ideal gas law as shown below. I find it hard to phrase my question. But the question is how come they were so confident that the relationship between the volume or pressure for an ideal gas law is going be stay linear until the very limit of absolute zero? One example comes to my mind of Hooke's Law. It is linear only to a certain limit therefore you just can't extrapolate the data. Could you please help me with it?
Source: http://www.passmyexams.co.uk/GCSE/physics/volume-temperature-relationship-of-gas-Charles-law.htmlHelpful links:
1: https://en.wikipedia.org/wiki/Absolute_zero#History
2: http://chemed.chem.purdue.edu/genchem/topicreview/bp/ch4/gaslaws3.html
3: https://en.wikipedia.org/wiki/Timeline_of_low-temperature_technology