chwala
Gold Member
- 2,827
- 415
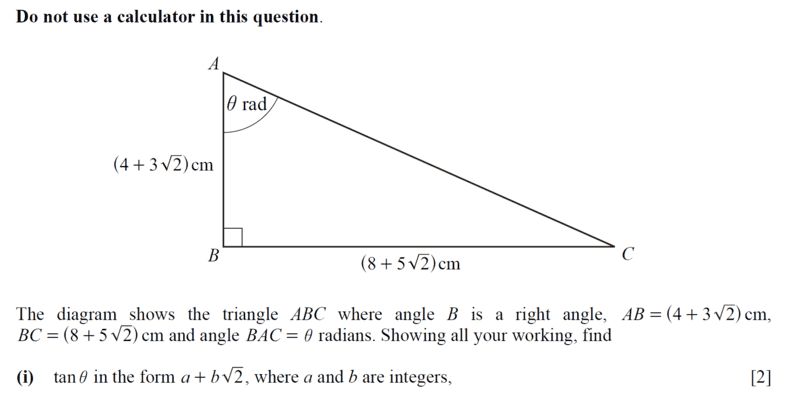
- Homework Statement
- see attached.
- Relevant Equations
- Trigonometry - Add Maths
There is an error on ms ...unless i am missing something.