Find the coefficient of friction and acceleration with masses given

Click For Summary

Homework Help Overview

The discussion revolves around a physics problem involving the calculation of the coefficient of friction and acceleration with given masses. Participants are exploring the relationships between energy, distance, and motion in the context of a system involving a suspended mass and a block on a table.

Discussion Character

  • Exploratory, Assumption checking, Problem interpretation

Approaches and Questions Raised

  • Participants discuss using energy methods to find acceleration but express concerns about the plausibility of the results. Questions are raised about the problem statement, particularly regarding the distance the suspended mass drops and its implications for calculations. There is also mention of constraints on the tools available for measuring the coefficient of friction.

Discussion Status

The discussion is active, with participants clarifying the problem setup and questioning assumptions about distances and measurements. Some guidance has been offered regarding the timing of the mass drop, which may help in calculating acceleration.

Contextual Notes

Participants note that they are limited to using a meter stick for measuring the coefficient of kinetic friction and that they were not provided with the acceleration directly. There is uncertainty about whether sufficient information is available to solve the problem effectively.

SSJBLOOD
Messages
5
Reaction score
0
Homework Statement
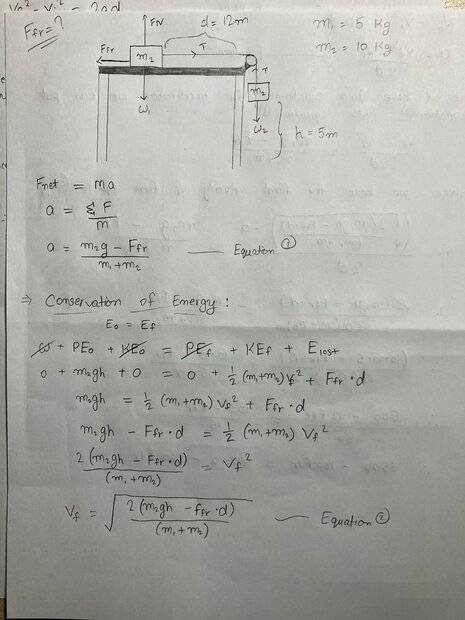
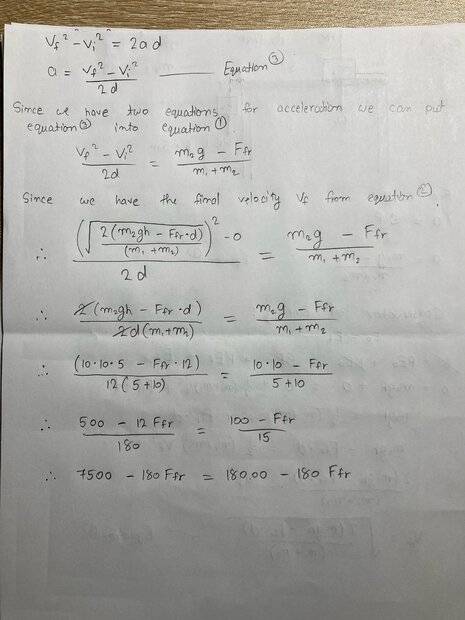
We need to find the kinetic friction and acceleration of a half Atwood system. The masses given are 295 grams and the smaller one is 147.5 grams.
Relevant Equations
\frac{1}{2}mv^{2}=mgh
\frac{g\left(m_{2}+m_{1}μ_{f}\right)}{m_{1}+m_{2}}
I tried to use the energy route but the acceleration was not a plausible one. It was just way too big.

new.jpeg
new2.jpeg
Image.jpeg
 
Physics news on Phys.org
What is the exact problem statement?
I see you are taking the distance for the friction as d=12m but the drop for the suspended mass is only 5m.
Since your KE takes the final velocity as applying to both masses, that should be when the upper mass has only moved 5m, no?
 
Oh I see now thanks a lot
 
I wasn't to sure on how to do it that was I just tried it
 
The only thing we were allowed to use was a meter stick to find the coefficient of kinetic friction between the block and the table surface. We weren't given the acceleration
 
SSJBLOOD said:
The only thing we were allowed to use was a meter stick to find the coefficient of kinetic friction between the block and the table surface. We weren't given the acceleration
You must know something else or there is not enough info. Did you time it until the suspended mass hit the floor? Or did the top mass stop before it reached the pulley and you measured how far it travelled in total?
 
Yeah we had time it was 3.23 seconds
 
SSJBLOOD said:
Yeah we had time it was 3.23 seconds
Then you can calculate the acceleration.
 
Reply
  • Like
Likes   Reactions: SSJBLOOD

Similar threads

Angular velocity- A toy train rolls around a horizontal track
  • · Replies 8 ·
Replies
8
Views
1K
How to find coefficient of friction of a body sliding down the slope?
  • · Replies 7 ·
Replies
7
Views
2K
Static Friction Between a Box and the Floor
  • · Replies 11 ·
Replies
11
Views
3K
Does coefficient of kinetic friction depend on speed?
  • · Replies 16 ·
Replies
16
Views
6K
Newton's Second Law (Pulley Sustem)
  • · Replies 3 ·
Replies
3
Views
2K
Finding the work done by a block
  • · Replies 4 ·
Replies
4
Views
3K
Why is the direction of kinetic/static friction up a ramp?
  • · Replies 7 ·
Replies
7
Views
2K
Friction Coefficients: Determining Force Direction?
  • · Replies 6 ·
Replies
6
Views
2K
Calculating Force and Acceleration in a Two Block System with Varying Friction
  • · Replies 6 ·
Replies
6
Views
1K
Having a hard time learning Newton's 2nd law
  • · Replies 5 ·
Replies
5
Views
2K