Find the exact shaded area of the region in 4 overlapping circles

[Zekes]

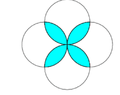
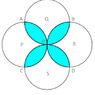
So, say you got 4 circles intersecting this way:

View attachment 9061

Now, I am looking for two things:

1. 
   A proof that each part of the circle which is in an intersection is 1/4 the size of the whole circle's circumference

• 
  The exact area of the non-shaded region.

Now, in my search to finding the answer to this, I stumbled upon this Circle-Circle Intersection -- from Wolfram MathWorld. The only problem? I have no idea what this article is trying to say, and how it can help me. I did find the equation to get the area of the shaded region ( it's $$A=2(\pi-2)$$ ) which I can use in Part 2 but I still don't understand how the solution got to there, and how to do Part 1. Please help me in learning what is trying to be said here in simpler terms! Thanks!

 

[Olinguito]

Hi Zekes.

Let the radius of each circle be $r$; let P, Q, R, S be the centres of the cirlces and O, A, B, C, D the points of intersections marked as follows:

View attachment 9063

Now PQRS is a square with diagonal $2r$ and so the length of each side is $r\sqrt2$, i.e. $|\mathrm{PQ}|=r\sqrt2$. So the “thickness” of each light-blue lens-shaped region of overlap between circles is $(2-\sqrt2)r$. if T is the point of intersection of the line segments AO and PQ, then

$$|\mathrm{PT}|\ =\ r-\frac{2-\sqrt2}2r\ =\ \frac r{\sqrt2}.$$

Hence $\angle\mathrm{APT}\ =\ \arccos\frac1{\sqrt2}\ =\ 45^\circ$; i.e. $\angle\mathrm{APO}=90^\circ$. That is to say, each circular arc drawn from O is one-quarter the circumference of each circle.

Now:

• area of quadrant APO = $\dfrac{\pi r^2}4$
  ;
• are of triangle APO = $\dfrac12r^2$
  ;
• therefore area of each light-blue shaded region = $2\times\left(\dfrac{\pi r^2}4-\dfrac12r^2\right)=\dfrac{(\pi-2)r^2}2$
  ;
• therefore area of non-shaded region in each circle = $\pi r^2-2\times\dfrac{(\pi-2)r^2}2=2r^2$
  ;
• therefore total non-shaded area = $4\times2r^2=8r^2$.

Interesting to note that the final answer does not contain $\pi$.