chwala
Gold Member
- 2,827
- 415
- Homework Statement
- see attached.
- Relevant Equations
- Mechanics
Now in determining the initial velocity;
in my understanding, if ##s=1.8## then we consider the stone's motion from the top to the ground. Why not consider ##s=3.6##, the total distance traveled by stone from start point ##t=0##? Is it possible to model equations from this point?
The stone when thrown upwards will reach a point where it is instantaneously at rest and then start the descent. In that case, it is clear that ##s=1.8##. I need insight on this very part.
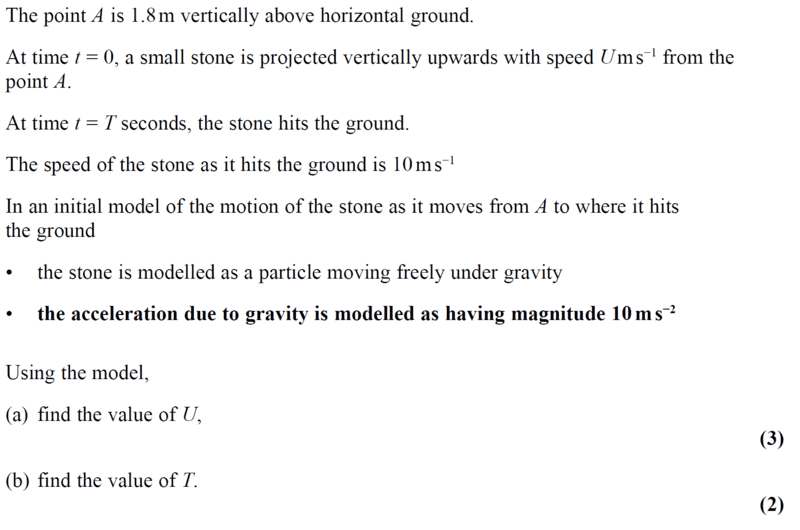

in my understanding, if ##s=1.8## then we consider the stone's motion from the top to the ground. Why not consider ##s=3.6##, the total distance traveled by stone from start point ##t=0##? Is it possible to model equations from this point?
The stone when thrown upwards will reach a point where it is instantaneously at rest and then start the descent. In that case, it is clear that ##s=1.8##. I need insight on this very part.
Last edited: