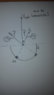
- #1
Gnall
- 18
- 1
- Homework Statement
- I have found the first following, I need b and c. The question is:
A total positive charge Q is uniformly distributed on an insulating thread of length L. The thread is bent into the shape of a semicircle and located in the xy plane, as shown in the figure. Express your answers in terms of the given quantities and fundemental constants as needed.
I found a following.
b.) If a charged particle with a mass m is located at the point x=0, y=L; the electric potential at P becomes zero. Find the particle's charge in terms of Q.
c.) If the charged particle at (b) is first carried to a point P, and then given an initial velocity v0 in the positive y direction, find the minimum value of v0 such that the particle escapes to a point infinitely far away from the charged thread. Ignore any gravitational effects.
- Relevant Equations
- Electric potential of semicircle at P point in the first following :
V= kQ/R
For the b following:
kQ/R=kq/L
L=πR
kQ/R=kq/πR
πQ=q
kQ/R=kq/L
L=πR
kQ/R=kq/πR
πQ=q