- #1
zehkari
- 22
- 3
- Homework Statement
- (See Attempt).
- Relevant Equations
- (See Attempt).
Hello all,
I have a question with the helix path of proton in a magnetic field that I am a bit stuck on.
Question:

Equations:
F = qv X B
F = mv^2/r
d=vt
My Attempt:
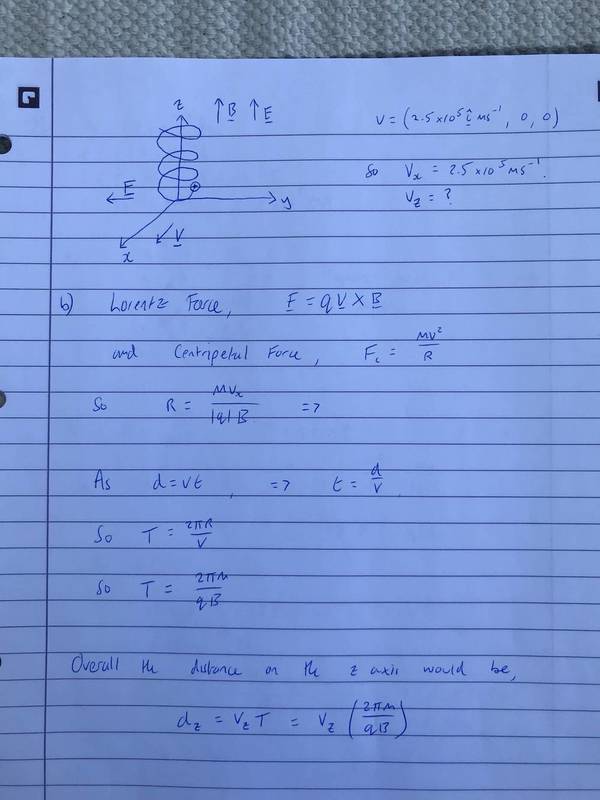
Think the graph drawn is good enough for questions (a). However, I am stuck on (b) and (c).
Firstly I am not entirely sure what question (b) is asking, the position where? At t = 0?
And then I can obtain an equation for the position of the proton after three complete revolutions (c), which is three 'pitches'. But I do not have the velocity in the z direction.
I also think I am overlooking the Electric field here. Which could play a role with the velocity in the z direction.
So I am not sure how to calculate the velocity in the z direction and what question (b) is asking.
Any help would be appreciated. Thank you for your time.
I have a question with the helix path of proton in a magnetic field that I am a bit stuck on.
Question:
Equations:
F = qv X B
F = mv^2/r
d=vt
My Attempt:
Think the graph drawn is good enough for questions (a). However, I am stuck on (b) and (c).
Firstly I am not entirely sure what question (b) is asking, the position where? At t = 0?
And then I can obtain an equation for the position of the proton after three complete revolutions (c), which is three 'pitches'. But I do not have the velocity in the z direction.
I also think I am overlooking the Electric field here. Which could play a role with the velocity in the z direction.
So I am not sure how to calculate the velocity in the z direction and what question (b) is asking.
Any help would be appreciated. Thank you for your time.