Find the Radius of 4th Circle When All are Tangent: Hint d/2

[Andrei1]

View attachment 741
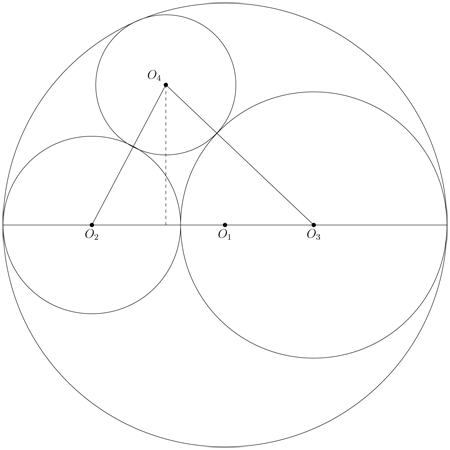
The centers of three circles are situated on a line. The center of the fourth circle is situated at given distance d from that line. What is the radius of the fourth circle if we know that each circle is tangent to other three. Please give me a hint, if you can. Answer: \(\displaystyle d/2.\)

 

[alyafey22]

Re: four circles

It is not clear what is d from the picture .

 

[mathmaniac1]

Re: four circles

It is not clear what is d from the picture .

The center of the fourth circle is situated at given distance d from that line.

I am also interested in the solution.

The center of the fourth circle is situated at given distance d from that line.

I am also interested in the solution.

Edit:

I also don't know where to start with this one.

But I really wonder why this works for all proportions in which the centre of the biggest circle is divided into.

It looks like d/2 is the radius of the 4th circle for all proportions (by my visualisation).

But to start working on it,I think I have to get to paper and pencil.

At first I would be looking for the case in which the centre of the big circle is divided into two equal parts as I assume it is easier to understand.

 

[Opalg]

The centers of three circles are situated on a line. The center of the fourth circle is situated at given distance d from that line. What is the radius of the fourth circle if we know that each circle is tangent to other three. Please give me a hint, if you can. Answer: \(\displaystyle d/2.\)

​

Write $r_1,\ r_2,\ r_3,\ r_4$ for the radii of the circles centred at $O_1,\ O_2,\ O_3,\ O_4$ respectively. Notice that $r_1 = r_2+r_3$. To calculate $r_4$ in terms of $r_2$ and $r_3$, use Descartes' theorem. For that, you need to use the curvatures $k_i = \pm1/r_i$ $(i=1,2,3,4)$. The first of these, $k_1$, will be negative (because the large circle touches the other three internally). Descartes' theorem says that $k_4 = k_1+k_2+k_3 \pm\sqrt{k_1k_2 + k_2k_3 + k_3k_1}$. When you substitute $k_1 = -1/(r_2+r_3)$, $k_i = 1/r_i$ for $i=2,3,4$, you find that the part inside the square root sign is zero (this corresponds to the fact that the centres $O_1,\ O_2,\ O_3$ are collinear). That gives you a formula for $r_4$ in terms of $r_2$ and $r_3$.

Now you have to bring in the distance $d$. I think the neatest way to do that is to use the fact that the area of triangle $O_2O_3O_4$ is $\frac12d(r_2+r_3)$. It is also given by Heron's formula in terms of $r_2,\ r_3,\ r_4$. Compare the two results and you will find that $r_4 = \frac12d$.

 

[CellCoree]

The radius of the fourth circle can be found by using the Pythagorean theorem and the given information. Since the centers of the three circles are on a line, we can draw a right triangle with one leg being the distance d and the other leg being the radius of the fourth circle. The hypotenuse of this triangle would be the sum of the radii of the three circles. Using the Pythagorean theorem, we can set up the equation (d/2)^2 + (r)^2 = (r + r + r)^2, where r is the radius of the fourth circle. Simplifying this equation, we get r = d/2. Therefore, the radius of the fourth circle is d/2.