Find the Ratio of BG to FG in a Figure with Given Side Lengths

[anemone]

Here is this week's POTW:

-----

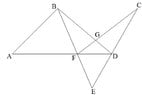
In the figure below, $AB=AF=15,\,FD=12,\,BD=18,\,BE=24$ and $CF=17$. Find \(\displaystyle \frac{BG}{FG}\).

https://www.physicsforums.com/attachments/5959._xfImport

-----

Remember to read the http://www.mathhelpboards.com/showthread.php?772-Problem-of-the-Week-%28POTW%29-Procedure-and-Guidelines to find out how to http://www.mathhelpboards.com/forms.php?do=form&fid=2!

 

[anemone]

No one answered last week problem. :(

You can see the proposed solution as follows:

Spoiler

By applying the Stewart's theorem on triangle $ABD$ and the line $BF$, we have:

$15^2\cdot 12+18^2 \cdot 15 =BF^2\cdot 27+15\cdot 12\cdot 27$

So $BF=10$ and so $EF=24-10=14$.

Again we use the Stewart's theorem on triangle $ABE$ and the line $AF$, we have:

$AE^2\cdot 10+15^2 \cdot 14 =15^2\cdot 24+14\cdot 10\cdot 24$

So $AE=\sqrt{561}$.

By Stewart's theorem on triangle $AED$ and the line $EF$, we have:

$ED^2\cdot 15+561 \cdot 12 =14^2\cdot 27+12\cdot 15\cdot 27$

So $ED=2\sqrt{57}$.

By Stewart's theorem on triangle $CFE$ and the line $FD$, we have:

$14^2\cdot CD+17^2 \cdot 2\sqrt{57} =12^2\cdot (CD+2\sqrt{57})+2\sqrt{57}\cdot 12\cdot CD \cdot (CD+2\sqrt{57})$

So $CD=\sqrt{57}$ and $CE=CD+DE=3\sqrt{57}$.

Note that $DG=18-BG$ and apply Menelaus' theorem to triangle $BED$ and the line through $C,\,G$ and $F$ to get \(\displaystyle 3\cdot\frac{18-BG}{BG}\cdot \frac{10}{14}=1\), so \(\displaystyle BG=\frac{135}{11}\).

Similarly, $CG=17-FG$, so applying Menelaus's theorem to triangle $CFE$ and the line through $B,\,G$ and $D$ we get \(\displaystyle \frac{24}{10}\cdot \frac{FG}{17-FG}\cdot \frac{1}{2}=1\) so \(\displaystyle FG=\frac{85}{11}\).

Therefore \(\displaystyle \frac{BG}{FG}=\frac{27}{17}\).