Find the scale factor of triangle ABC to triangle DEF

[masters1]

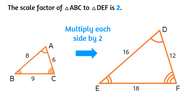
Triangles ABC and DEF are similar.

Triangle ABC has a perimeter of 16cm.

Triangle DEF has side of 6cm, 8cm and 10cm.

What is the scale factor of triangle ABC to triangle DEF?

A. 1/2
B. 1/3
C. 2/3
D. 3/2
E. 2/1

I concluded the answer is D. Am I correct?

 

[skeeter]

What is the scale factor of triangle ABC to triangle DEF?

perimeter of ABC : perimeter of DEF = 16:24 = 2:3

 

[HallsofIvy]

There is a difference between the "scale factor of triangle ABC to triangle DEF" and the "scale factor of triangle DEF to triangle ABC". You found the wrong one! Since ABC is smaller than DEF, the scale factor is less than 1.

 

[masters1]

Then, is this textbook example incorrect?

 

[skeeter]

The dilation scale factor, which is a transformation, of triangle ABC to triangle DEF is 3/2

The scale ratio, like a map's scale, of triangle ABC to triangle DEF is 2/3

Sorry for the confusion.

 

[masters1]

The dilation scale factor, which is a transformation, of triangle ABC to triangle DEF is 3/2

The scale ratio, like a map's scale, of triangle ABC to triangle DEF is 2/3

Sorry for the confusion.

So, the question asked about "scale factor", not ratio of any kind. Is the answer, in fact, "D", which is 3/2?