chwala
Gold Member
- 2,827
- 415
- Homework Statement
- see attached
- Relevant Equations
- ode/pde
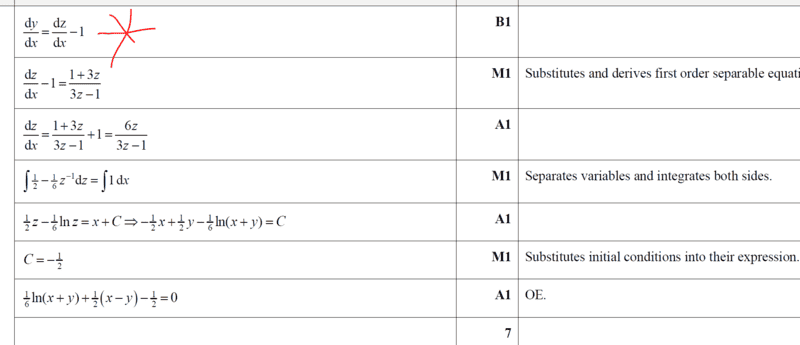
I need insight on the highlighted in Red on how ##\left[\dfrac{dz}{dx} - 1 = \dfrac{dy}{dx}\right]## otherwise the rest of the steps are clear. I just read that ##\dfrac{dx}{dy} \dfrac{dy}{dz} \dfrac{dz}{dx} =-1##