- #1
mathlearn
- 331
- 0
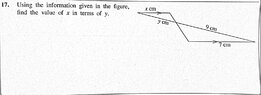
View attachment 7558
All I see is the three angles of the triangles equal.
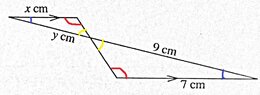
View attachment 7559
Any Ideas on how to begin ?
Many Thanks :)
All I see is the three angles of the triangles equal.
View attachment 7559
Any Ideas on how to begin ?
Many Thanks :)
Attachments
Last edited by a moderator: