tixi
- 4
- 3
- Homework Statement
- The function ln (principal value of ln) is known to be analytical in complex plane with removed negative real axis. What is the largest region in which f(z)=ln(iz)-i pi/2 is analytical? Evaluate f′(z).
- Relevant Equations
- Principal value of the logarithm: ln(z) = ln(r) + iArg(z)
Chain rule for complex functions
Inverse functions differentiation rule
Hey everyone! I got stuck with one of my homework questions. I don't 100% understand the question, let alone how I should get started with the problem.
The picture shows the whole problem, but I think I managed doing the a and b parts, just got stuck with c. How do I find the largest region in which f(z) is analytical and how do I get started trying to differentiate it? Do the differentiation rules for the real ln translate to the complex one?
Thanks in advance <3
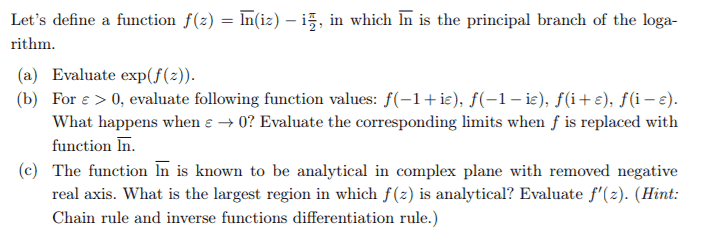
The picture shows the whole problem, but I think I managed doing the a and b parts, just got stuck with c. How do I find the largest region in which f(z) is analytical and how do I get started trying to differentiate it? Do the differentiation rules for the real ln translate to the complex one?
Thanks in advance <3