Eobardrush
- 18
- 3
- Homework Statement
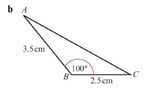
- Find the area of this triangle
- Relevant Equations
- 0.5 x a x c x Sin B
I just simply used the formula to solve. Note the "x" represents multiplication in this case
0.5 x a x c Sin B
This is based on the conditions given in the textbook I am using which quotes "Use this formula to find the area of any triangle when you know 2 sides and an angle between them"
So I got my answer as follows: 0.5 x 3.5 x 2.5 x Sin 100 = 4.31 cm2
It is correct but what I am struggling is in understanding how I can do this without relying on the formula, like splitting this into a right angled triangle(by finding height for example)
I attached a diagram I drew about this triangle. As you can see I tried to split this into a right angled triangle
Can someone give me some insights on how I can try to find this without relying on the formula so that I would better understand how this works?
Note that I did see the proof of the formula but it was for the normal looking triangles(with acute angles) so I am not sure how to apply the proof to this type of triangle
0.5 x a x c Sin B
This is based on the conditions given in the textbook I am using which quotes "Use this formula to find the area of any triangle when you know 2 sides and an angle between them"
So I got my answer as follows: 0.5 x 3.5 x 2.5 x Sin 100 = 4.31 cm2
It is correct but what I am struggling is in understanding how I can do this without relying on the formula, like splitting this into a right angled triangle(by finding height for example)
I attached a diagram I drew about this triangle. As you can see I tried to split this into a right angled triangle
Can someone give me some insights on how I can try to find this without relying on the formula so that I would better understand how this works?
Note that I did see the proof of the formula but it was for the normal looking triangles(with acute angles) so I am not sure how to apply the proof to this type of triangle
Attachments
Last edited: