- #1
markosheehan
- 136
- 0
View attachment 5960
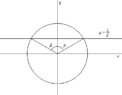
can some one help me with part b finding the co-ordinates of p. i tried this by letting sin 2x=1/2 but when i work out x i do not get the right answer. the right answer is (17pi/12, 1/2)
can some one help me with part b finding the co-ordinates of p. i tried this by letting sin 2x=1/2 but when i work out x i do not get the right answer. the right answer is (17pi/12, 1/2)