Finding crossing regions between two quadractics

[rockerman]

Given two quadratics of the form $f(x) = x'Q_1x$ and $g(x) = x'Q_2x$ and assuming $Q_1$ and $Q_2$ are negative definite matrices, how can I find the lines that are formed by their crossing? here I'm assuming $x \in \mathbb{R}^2$.

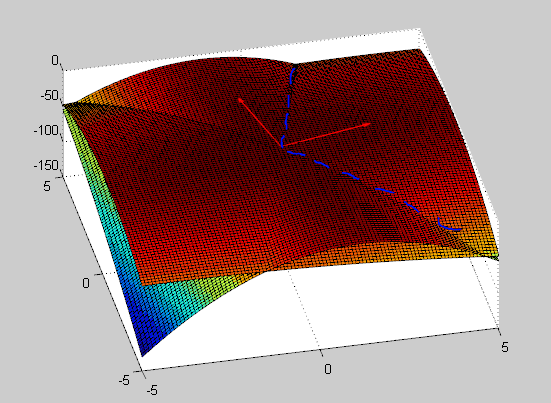
I'm interested in finding vectors $w_1$ and $w_2$ that are parallel to the blue dashed line. I also outlined the eigenvectors of $Q_1-Q-2$, they seem to point exact in the middle of each region.

 

[andrewkirk]

Write x as (x y)' and label the eight (known) entries of the two matrices, then write out $x'(Q_1-Q_2)x=0$ as an expansion that will be of the form $\alpha x^2+\beta xy+\gamma y^2$. Then solve for $\frac{x}{y}$.