- #1
CallMeShady
- 45
- 1
Now let me start off by saying that I, along with over 100 students in my class are having issues with this concept, thanks to our "amazing" professor, while our midterm exam is a few days away. I don't want to sound like I am complaining, but I am frustrated with the fact that the professor refuses to go over an example with us completely. The following is a practice exam question and for some reason, he refuses to provide answers to the questions (forget providing an answer key). Any help would be greatly appreciated.
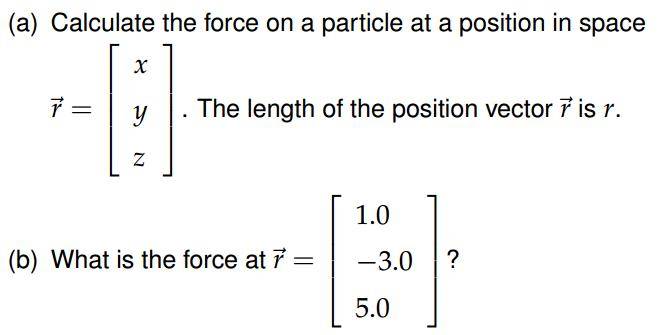
V(r; C6, C12) = C12/r12 - C6/r6 with C12 = 1.0 and C6 = 2.0
None, all equations you need for the question are in the question itself.
So I know that F(vector) = -∇V, where F is the force and V is the potential energy. Basically this means that I need to take the partial derivative of V(r) and multiply that by r(vector)/r and so for part (a), I get: (12C12r-13-6C6r-7)(r(vector)/r)
Have I done this correct? And if so, how do I proceed to part (b)?
Homework Statement
V(r; C6, C12) = C12/r12 - C6/r6 with C12 = 1.0 and C6 = 2.0
Homework Equations
None, all equations you need for the question are in the question itself.
The Attempt at a Solution
So I know that F(vector) = -∇V, where F is the force and V is the potential energy. Basically this means that I need to take the partial derivative of V(r) and multiply that by r(vector)/r and so for part (a), I get: (12C12r-13-6C6r-7)(r(vector)/r)
Have I done this correct? And if so, how do I proceed to part (b)?