Finding gauge pressure for gas inside light bulb as it heats up

[member 731016]

Homework Statement

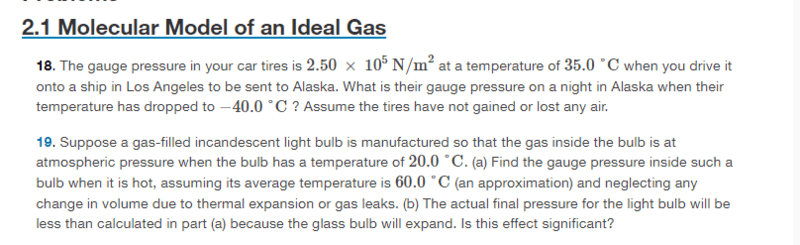
Suppose a gas-filled incandescent light bulb is manufactured so that the gas inside the bulb is at atmospheric pressure when the bulb has a temperature of 20.0°C. (a) Find the gauge pressure inside such a bulb when it is hot, assuming its average temperature is 60.0°C (an approximation) and neglecting any change in volume due to thermal expansion or gas leaks. (b) The actual final pressure for the light bulb will be less than calculated in part (a) because the glass bulb will expand. Is this effect significant?

Relevant Equations

PV = nRT

For this 19(a),

The answer is 0.137 atm.

My working is
$P_{gauge} = P_f - P_i$
$P_{gauge} = \frac{nRT_f}{V_f} - \frac{nRT_i}{V_i}$
$P_{gauge} = \frac{nRT_f}{V} - \frac{nRT_i}{V}$ since volume does not change
$P_{gauge} = \frac{nR}{V}(T_f - T_i)$

However, I am not sure how to go from here since we do not know the constant volume. Does someone please know how to tackle this?

Many thanks!

 

[haruspex]

There is a piece of information you have not used. Can you spot it?

 

[member 731016]

There is a piece of information you have not used. Can you spot it?

Thank you for your reply @haruspex!

No sorry, I cannot spot it.

Many thanks!

 

[haruspex]

"the gas inside the bulb is at atmospheric pressure when the bulb has a temperature of 20.0°C"

 

[member 731016]

"the gas inside the bulb is at atmospheric pressure when the bulb has a temperature of 20.0°C"

Ah, thank you for your reply @haruspex!

I see how the volume of the gas can be found now :)

 

[Chestermiller]

$$\frac{P_f}{P_i}=\frac{T_f}{T_i}$$

 

[member 731016]

$$\frac{P_f}{P_i}=\frac{T_f}{T_i}$$

Thank you for your reply @Chestermiller !