JohnnyLaws
- 10
- 0
- Homework Statement
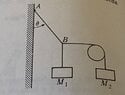
- We have two bodies (first one has 300Kg and another one has400Kg) in equilibrium connected by a massless string and there's no friction. The first one is held by a thread that makes an angle theta with the wall as we will see in image. The second one is held by same thread that is held by a pulley. I need to calculate angle theta and the Tension that string makes between points A and B (points that We Will see in image).
- Relevant Equations
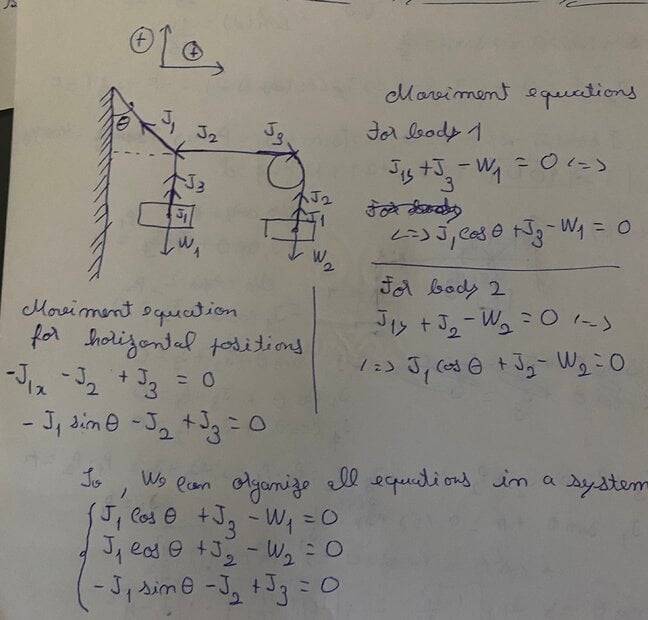
- To define the equations I used (I'm not sure if they are correct), I need to explain force vectors. It's probably better to start by looking at the image before we delve into the equations.
T1 = is first Tension wich is produzed in points A and B.
T2 = is Tension that body 2 creates
T3 = is Tension that body creates
W1 = weight of body 1
W2 = weight of body 2
T1cos(theta)+T3-W1 = 0
That first equation is refering to body 1
T1cos(theta)+T2-w2 = 0
That second equation is refering to body 2
-T1sin(theta)-T2+T3= 0
That thirth equation is refering to body horizontal forces of the string
I'm pretty sure those equations are wrong because I have 3 variables for 4 equations
For a better understanding of this exercise here is the image illustrating the scenario described in the statement:
View attachment 330011
So to solve this exercise I began by drawing a forces diagram:
I believe I have explained everything in the "Relevant equations" section. What am I doing wrong? The book that I'm reading doesn't have any solutions.
View attachment 330011
So to solve this exercise I began by drawing a forces diagram:
I believe I have explained everything in the "Relevant equations" section. What am I doing wrong? The book that I'm reading doesn't have any solutions.