Finding the direction of infinite limits

[Weightlifting]

Homework Statement

Find the vertical asymptote of $y=\frac{2x-5}{\left|3x+2\right|}$. If there is an infinite limit, what direction does it go in?

Relevant Equations

Note: I'm currently only at the stage of using the informal definition of limits ("Calculus, a complete course" by Robert A. Adams, chapter 1.3, 9th edition, problem 34, to be exact), so I'm looking for help without using the epsilon-delta method

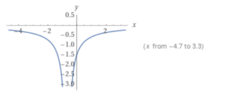
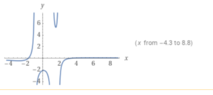
Since $\left|3x+2\right|=0\rightarrow\ x=-\frac{2}{3}$, we know the vertical asymptote is at $x=-\frac{2}{3}$.

Looking at the limit at that point, and also looking at the left- and right-sided limit, I cannot simplify it any further: $\lim_{x\rightarrow-\frac{2}{3}}{\frac{2x-5}{\left|3x+2\right|}}=\lim_{x\rightarrow-\frac{2}{3}\pm}{\frac{2x-5}{\pm(3x+2)}}$.

That being said, is there an easy way to find out whether the limit is going towards infinity, and which direction the infinity goes? Or should I be expected to be able to quickly visualize what a graph of a function looks like, and determine from there which direction the graph goes? Right now, I just plotted it using computer software to see that the direction of the limit goes towards negative infinity, see attachment. However, Is there a method to quickly determine this without using software?

Thank you!

 

[Weightlifting]

Sorry, first time using LaTeX, but I cannot edit my HW statement anymore it seems. It should be:

Find the vertical asymptote of $y=\frac{2x-5}{\left|3x+2\right|}$. If there is an infinite limit, what direction does it go in?

 

[PeroK]

Sorry, first time using LaTeX, but I cannot edit my HW statement anymore it seems. It should be:

Find the vertical asymptote of $y=\frac{2x-5}{\left|3x+2\right|}$. If there is an infinite limit, what direction does it go in?

You can just try $x$ slightly less than and slightly greater than $-\frac 2 3$.

 

[Weightlifting]

You can just try $x$ slightly less than and slightly greater than $-\frac 2 3$.

Hi PeroK, thanks for your input!

However, my problem with this is, how close should I go, because $y<0$ for $x<\frac{5}{2}$, but after that, it's greater than 0. How do I know how close I should go to $x=-\frac{2}{3}$?

 

[PeroK]

Hi PeroK, thanks for your input!

However, my problem with this is, how close should I go, because $y<0$ for $x<\frac{5}{2}$, but after that, it's greater than 0. How do I know how close I should go to $x=-\frac{2}{3}$?

In the context of a vertical asymptote, $\frac 5 2$ is long way from $-\frac 2 3$.

 

[Weightlifting]

Hi PeroK, thanks for your input!

However, my problem with this is, how close should I go, because $y<0$ for $x<\frac{5}{2}$, but after that, it's greater than 0. How do I know how close I should go to $x=-\frac{2}{3}$?

For example, lets take $y=\frac{x}{\left(x-1\right)^2}$. for $x<-1$, the graph seems to go to negative infinity, while it't only between $-1<x<1$ that the graph goes up again. Of course this is still relatively straightforward, but the tougher the equation goes, the harder it would be for me to figure this out. So I'm looking for a general rule, or some kind of missing logic here.

 

[Weightlifting]

In the context of a vertical asymptote, $\frac 5 2$ is long way from $-\frac 2 3$.

Lets just do the more 'extreme' example, I could relatively easily figure out where the vertical asymptotes of $y=\frac{x^4-3x^3+3x-19}{x^5-9x^2+9}$ would be, but how would I know which direction they would tend to for each asymptote?

 

[FactChecker]

Looking at the limit at that point, and also looking at the left- and right-sided limit, I cannot simplify it any further: $\lim_{x\rightarrow-\frac{2}{3}}{\frac{2x-5}{\left|3x+2\right|}}=\lim_{x\rightarrow-\frac{2}{3}\pm}{\frac{2x-5}{\pm(3x+2)}}$.

Replacing $|3x+2|$ with $\pm(3x+2)$ is a bad step if you take it literally. The $\pm$ means that the denominator can be positive or negative, but you know that $|3x+2|$ is always positive. You should not lose track of that.
Because the denominator, $|3x+2|$, is always positive, you know that the sign of the original fraction will be the same as the sign of the numerator at $x=-2/3$. It is $2 (-2/3) - 5 = -19/3 \lt 0$.

 

[PeroK]

For example, lets take $y=\frac{x}{\left(x-1\right)^2}$. for $x<-1$, the graph seems to go to negative infinity, while it't only between $-1<x<1$ that the graph goes up again. Of course this is still relatively straightforward, but the tougher the equation goes, the harder it would be for me to figure this out. So I'm looking for a general rule, or some kind of missing logic here.

Most cases should be straightforward, like this one. The only complication comes if the numerator changes sign close to the asymptote. And, where the zeros of the numerator are not easy to calculate. In those cases, which you are unlikely to face, you'll have to rely on your wits and numeracy skills.

 

[FactChecker]

Most cases should be straightforward, like this one. The only complication comes if the numerator changes sign close to the asymptote. And, where the zeros of the numerator are not easy to calculate. In those cases, which you are unlikely to face, you'll have to rely on your wits and numeracy skills.

To be precise, you are unlikely to see hard cases in typical homework problems. The real world can be messier. One thing that you might run into in homework is the situation where both numerator and denominator have zeros at the same x value. The class will probably explain what to do in those cases.

 

[PeroK]

One thing that you might run into in homework is the situation where both numerator and denominator have zeros at the same x value. The class will probably explain what to do in those cases.

Then there is possibly no asymptote!