Finding the inverse of 4th rank elasticity tensor

In summary, the process of finding the inverse of a 4th rank elasticity tensor involves applying mathematical techniques specific to tensor analysis, which includes manipulating the components of the tensor to derive its inverse. This is crucial in material science and engineering for understanding the relationship between stress and strain in materials. The process typically requires the use of determinant and cofactor methods, or more advanced numerical techniques, to ensure accurate calculations for complex materials where elasticity properties vary.
  • #1
Pilou115
1
0
Homework Statement
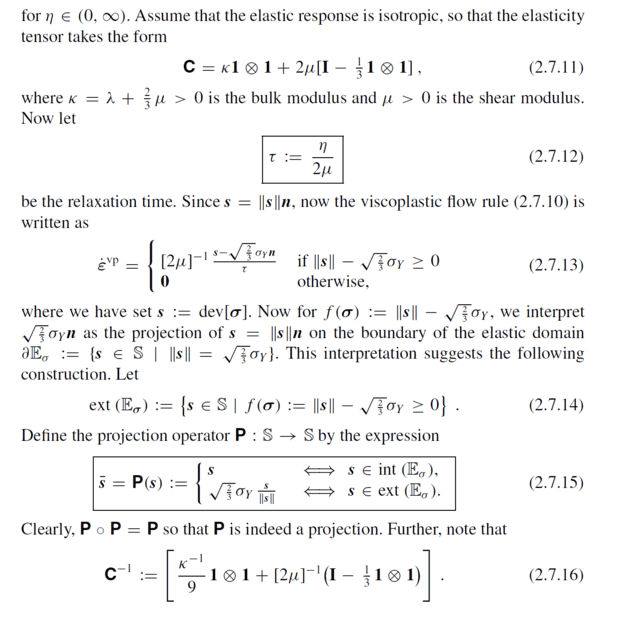
I need to find how to obtain the expression of the inverse of a rank four elasticity tensor.
Relevant Equations
C = k 1x1 + 2µ[I-1/3*1x1] where C in the foutrth order tensor
C^-1 = k^(-1)/9 1x1 + 2µ^(-1)[I-1/3*1x1]
I'm desperately trying to understand how to get from 2.7.11 to 2.7.16 and cannot find any reference online on how to find the inverse of an elastic tangent modulus (fourth_order tensor). Can someone help me or give me a reference I can check where they do a similar thing? I would really appreciate it !

1712785536404.png



 
Physics news on Phys.org
  • #2
If you know how to do tensor algebra, you should be able to show that ##C/otimes C^{-1}=\text{ the identity tensor.}## (I don't know how to do it.)
 

FAQ: Finding the inverse of 4th rank elasticity tensor

What is a 4th rank elasticity tensor?

A 4th rank elasticity tensor is a mathematical representation used in continuum mechanics to describe the relationship between stress and strain in a material. It is a fourth-order tensor that relates the components of stress to the components of strain in three-dimensional space, capturing the material's elastic properties, including anisotropy and symmetry.

Why do we need to find the inverse of a 4th rank elasticity tensor?

Finding the inverse of a 4th rank elasticity tensor is crucial for determining the material's compliance tensor, which describes how the material deforms under applied stresses. The inverse relationship allows engineers and scientists to analyze material behavior under various loading conditions, facilitating the design and optimization of structures and materials.

How is the inverse of a 4th rank elasticity tensor calculated?

The inverse of a 4th rank elasticity tensor can be calculated using tensor algebra, often involving the use of matrix representation. The tensor can be expressed in a matrix form, and standard matrix inversion techniques can be applied. However, care must be taken to account for the tensor's specific symmetries and properties, as these can complicate the inversion process.

What challenges are associated with inverting a 4th rank elasticity tensor?

Challenges in inverting a 4th rank elasticity tensor include dealing with its complexity, as the tensor has 81 components in three-dimensional space, many of which may be zero or related due to symmetries. Additionally, numerical stability and precision can be issues, especially for tensors that are close to singular or ill-conditioned. Proper mathematical techniques and software tools are often required to ensure accurate results.

What applications rely on the inverse of a 4th rank elasticity tensor?

Applications that rely on the inverse of a 4th rank elasticity tensor include materials science, structural engineering, geophysics, and biomechanics. In these fields, understanding material deformation and behavior under stress is essential for designing safe and efficient structures, analyzing geological formations, and studying biological tissues.

Similar threads

Constraints on a fourth rank tensor
Replies
16
Views
2K
Finding the inverse metric tensor from a given line element
Replies
2
Views
2K
GR: Metric, Inverse Metric, Affine Connection Caluculation Help
Replies
1
Views
3K
Questions from Quantum Measurements
Replies
12
Views
2K
Classical Books about tensor analysis just good enough for physics
Replies
5
Views
2K
B Are Inverse Problems More Complex Than Direct Problems in Mathematics?
Replies
13
Views
2K
A Product of 3rd rank tensor with squared vector
Replies
9
Views
4K
A 4th order tensor inverse and double dot product computation
Replies
1
Views
4K
Understanding elastic tensor matrix intuitively
Replies
4
Views
2K
How can I calculate the inverse of a 4th order tensor?
Replies
5
Views
7K

Hot Threads

Recent Insights

Back
Top