karush
Gold Member
MHB
- 3,240
- 5
Ray $\overline{PK}$ bisects and the measure of $\angle{LPM}$ is $11x^o$ and the measure of $\angle{LPK}$ is $(4x+18)^o$
What is the measure of
$\angle{KPM}$
$s.\ 12^o \quad b.\ 28\dfrac{2}{7}^o \quad c. \ 42^o \quad d. \ 61\dfrac{1}{5}^o \quad e. \ 66^o$
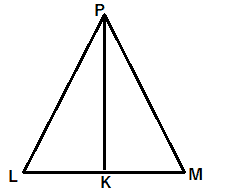
What is the measure of
$\angle{KPM}$
$s.\ 12^o \quad b.\ 28\dfrac{2}{7}^o \quad c. \ 42^o \quad d. \ 61\dfrac{1}{5}^o \quad e. \ 66^o$
Last edited: