- #1
happyparticle
- 456
- 21
- Homework Statement
- Finding when the oscillations of the mass A will be at the minimum amplitude for the first time.
At t = 0, pendulum A = ##x_a = 5cm## and pendulum B = ##x_a = 0##
- Relevant Equations
- normal modes coordinates
##q_p(t) = A cos \omega_p t##
##q_b(t) = B cos \omega_b t##
First of all, I found the angular frequencies for both pendulum and breathing mode which are
##\omega_p = 4.95##
##\omega_b = 7.45##
Then I found the normal mode coordinates equations:
##q_p(t) = A cos \omega_p t##
##q_b(t) = B cos \omega_b t##
And the beating frequency (I'm not sure if I need it or even if the value is correct)
##f_{beat} = 0.4##
Because the initial velocities are zero, the amplitude of each normal mode is equal to the initial value of the normal mode coordinate.
The pendulum A is at ##x_a = 5## and because the pendulum B is at ##x_a = 0## the initial position of the pendulum a (I guess)##q_p = x_1 + x_2, q_b = x_1 - x_2##
##q_p = 5 + ?, q_b = 5 - ?##
then
##q_p(t) = (5 + ?) cos \omega_p t##
##q_b(t) = (5-?) cos \omega_b t##
I know as well that
##x_1 = \frac{q_p(t) + q_b(t)}{2}##
##x_2 = \frac{q_p(t) - q_b(t)}{2}##
I'm stuck there. I still not sure about ##x_2## position and I can't see when the oscillations of the pendulum A will be at the minimum amplitude for the first time.
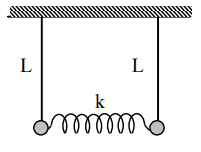
##\omega_p = 4.95##
##\omega_b = 7.45##
Then I found the normal mode coordinates equations:
##q_p(t) = A cos \omega_p t##
##q_b(t) = B cos \omega_b t##
And the beating frequency (I'm not sure if I need it or even if the value is correct)
##f_{beat} = 0.4##
Because the initial velocities are zero, the amplitude of each normal mode is equal to the initial value of the normal mode coordinate.
The pendulum A is at ##x_a = 5## and because the pendulum B is at ##x_a = 0## the initial position of the pendulum a (I guess)##q_p = x_1 + x_2, q_b = x_1 - x_2##
##q_p = 5 + ?, q_b = 5 - ?##
then
##q_p(t) = (5 + ?) cos \omega_p t##
##q_b(t) = (5-?) cos \omega_b t##
I know as well that
##x_1 = \frac{q_p(t) + q_b(t)}{2}##
##x_2 = \frac{q_p(t) - q_b(t)}{2}##
I'm stuck there. I still not sure about ##x_2## position and I can't see when the oscillations of the pendulum A will be at the minimum amplitude for the first time.
Last edited by a moderator: