Finding the projection of a Vector

In summary, the conversation discusses a possible mistake in a highlighted formula and the confusion between a vector unit and imaginary unit. The person is seeking confirmation and mentions the need for a better resource if there is indeed a mistake.
  • #1
chwala
Gold Member
2,697
369
Homework Statement
Kindly see attached.
Relevant Equations
vector knowledge
I am looking at this now; pretty straightforward as long as you are conversant with the formula: anyway i think there is a mistake on highlighted i.e
1689563985799.png
1689564008049.png
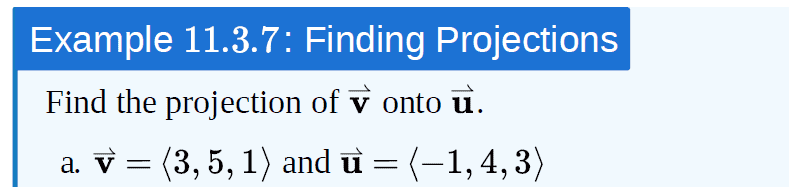
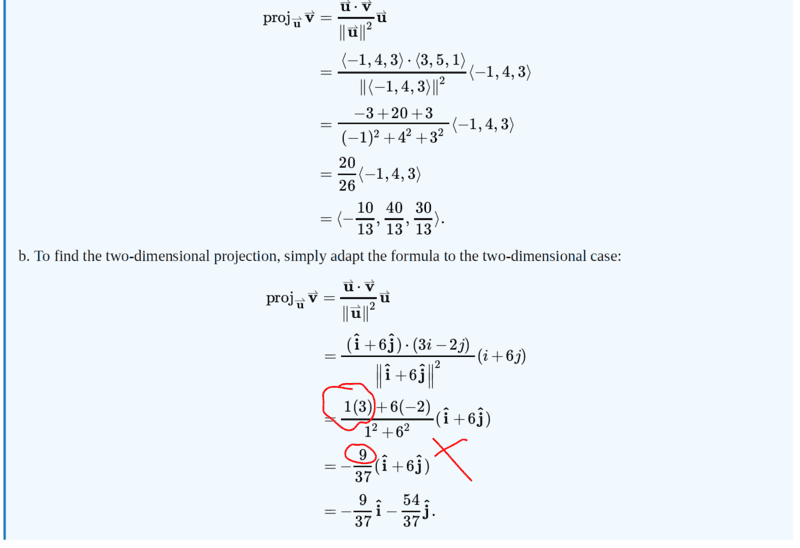Ought to be

##-\dfrac{15}{37}(i+6j)##

just need a confirmation as at times i may miss to see something. If indeed its a mistake then its time to look for a better resource.
 
Last edited:
Physics news on Phys.org
  • #2
##1(3) + 6(-2) = 3 - 12 = -9##

Note the negative sign out front of the fraction.

-Dan
 
  • Like
Likes chwala
  • #3
Maybe you are confusing a vector unit ##\mathbf{i}## with imaginary unit i.
 
  • Like
Likes chwala
  • #4
topsquark said:
##1(3) + 6(-2) = 3 - 12 = -9##

Note the negative sign out front of the fraction.

-Dan
but

##\vec u= -i+4j+3k##

aaaaaargh let me take a break...thanks man.
 
  • #5
anuttarasammyak said:
Maybe you are confusing a vector unit ##\mathbf{i}## with imaginary unit i.
Actually, i was looking at the wrong question...you realise that i have posted a: question which is referenced to a totally different solution i.e b: solution.
 

Related to Finding the projection of a Vector

1. What is the projection of a vector?

The projection of a vector is a vector that represents the shadow or image of one vector onto another vector. It is essentially the component of one vector in the direction of another vector.

2. How do you calculate the projection of vector A onto vector B?

The projection of vector A onto vector B is calculated using the formula: \[ \text{proj}_B(A) = \left( \frac{A \cdot B}{B \cdot B} \right) B \]where \( A \cdot B \) is the dot product of vectors A and B, and \( B \cdot B \) is the dot product of vector B with itself.

3. What is the geometric interpretation of the projection of a vector?

Geometrically, the projection of a vector A onto vector B is the orthogonal projection of A onto a line parallel to B. It represents how much of vector A is in the direction of vector B.

4. What are some applications of vector projection?

Vector projection is used in various fields such as physics, engineering, and computer graphics. Applications include decomposing forces in mechanics, calculating work done by a force, and determining the alignment or similarity between vectors in machine learning and data analysis.

5. Can the projection of a vector be longer than the original vector?

No, the projection of a vector onto another vector cannot be longer than the original vector. The length of the projection is always less than or equal to the length of the original vector, as it represents only the component of the vector in the direction of the other vector.

Similar threads

Vectors & Angles Problem Analysis
Replies
2
Views
1K
Cauchy's Formula for Stress tensor
Replies
12
Views
1K
Projection of vector vs vector components
Replies
8
Views
5K
Kinematics, curvilinear motion (a couple of questions)
Replies
30
Views
827
Problem involving tangent vector, normal vector, binormal vector and curvature
Replies
2
Views
4K
Understanding the Usefulness of Vector Projection
Replies
12
Views
5K
I Verify a Formula for Poynting Vector?
Replies
3
Views
1K
Studying High-Functioning Autistic Adult - how to find graduate tutors?
Replies
23
Views
1K
What is the formula for calculating the arclength of a general curve?
Replies
1
Views
2K
Electronics Switches, Pots, Displays for DIY projects - Some ideas, some questions
Replies
6
Views
3K

Hot Threads

Recent Insights

Back
Top