Finding the time for an object to start rolling without slipping

[member 731016]

Homework Statement

I am trying to derive the equation that my textbook presents (5.28), however, I notice that they don't use the right hand rule for the torque so there is a slight change in the sign in my derivation

Relevant Equations

$v_r = r\omega$ for is a condition for rolling without slipping where $v_r$ is the speed of the COM of the object

For this,

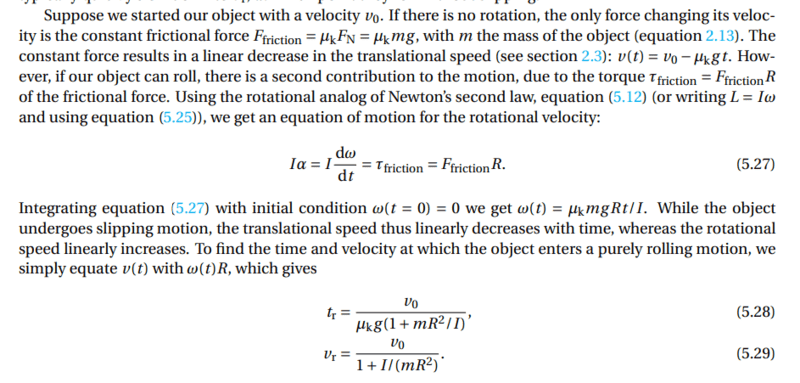
I don't understand why they don't have a negative sign as the torque to the friction should be negative. To my understanding, I think the equation 5.27 should be $I\frac{d \omega}{dt} = -F_{friction}R$ from the right hand rule assuming out of the page is positive.

Noting that $f_k = \mu_kmg$ and integrating both sides, I get the equation of motion $\frac{-Rmg \mu_kt}{I} = \omega(t)$

I also get $v(t) = v_0 - u_kgt$

So setting the two equations equal to each other in the relation for rolling motion:

$v(t) = R \omega (t)$

I get $t_r = \frac{v_0}{\mu_kg(1 - \frac{mR^2}{I})}$. Could someone please explain to me who is wrong and why?

Many thanks!

 

[haruspex]

assuming out of the page is positive.

What you quote of the problem does not indicate whether the motion is left to right or right to left. Assuming usual conventions and a positive velocity, it is L to R. In that case, the angular acceleration is clockwise, so negative and into the page.
Correspondingly, at rolling, $v=-R\omega$.

 

[member 731016]

What you quote of the problem does not indicate whether the motion is left to right or right to left. Assuming usual conventions and a positive velocity, it is L to R. In that case, the angular acceleration is clockwise, so negative and into the page.
Correspondingly, at rolling, $v=-R\omega$.

Thank you for your reply @haruspex! However, do you please know where the equation $v = -R \omega$ came from? Sorry I have not seen that equation with the minus sign before (I have only seen $v = R \omega$)

Many thanks!

 

[haruspex]

Thank you for your reply @haruspex! However, do you please know where the equation $v = -R \omega$ came from? Sorry I have not seen that equation with the minus sign before (I have only seen $v = R \omega$)

Many thanks!

The commonest convention is positive to the right for velocity and positive anticlockwise for rotation. If a disc is rolling to the right along a line underneath it then its rotation is clockwise, so a positive velocity means a negative rotation, etc.
You can think of it as being because the radius, measured from the axis to the ground contact is downwards, so negative. If it were rolling along the ceiling then there would be no minus sign.

 

[member 731016]

The commonest convention is positive to the right for velocity and positive anticlockwise for rotation. If a disc is rolling to the right along a line underneath it then its rotation is clockwise, so a positive velocity means a negative rotation, etc.
You can think of it as being because the radius, measured from the axis to the ground contact is downwards, so negative. If it were rolling along the ceiling then there would be no minus sign.

Thank you for your help @haruspex! Your explanation makes sense