Finitely Generated Modules and Maximal Submodules

[Math Amateur]

I am reading Paul E. Bland's book, "Rings and Their Modules".

I am focused on Section 6.1 The Jacobson Radical ... ...

I need help with an aspect of Proposition 6.1.2 ... ...

Proposition 6.1.2 relies on Zorn's Lemma and the notion of inductive sets ... ... so I am providing a short note from Bland on Zorn's Lemma and inductive sets ... ... as follows:

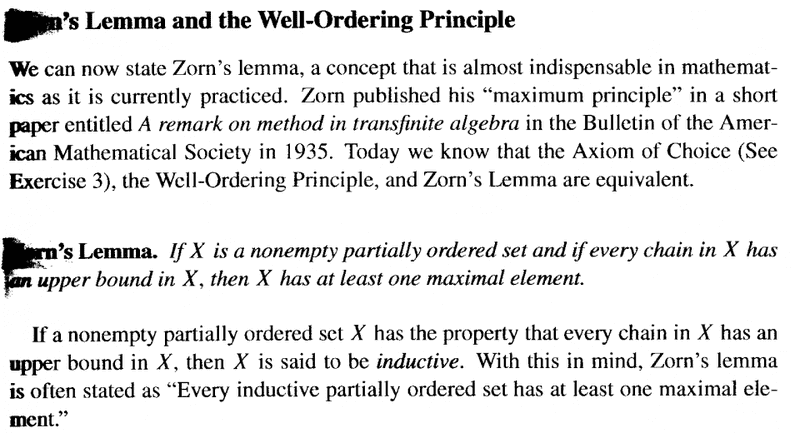
NOTE: My apologies for the poor quality of the above image - due to some over-enthusiastic highlighting of Bland's text

Now, Proposition 6.1.2 reads as follows:

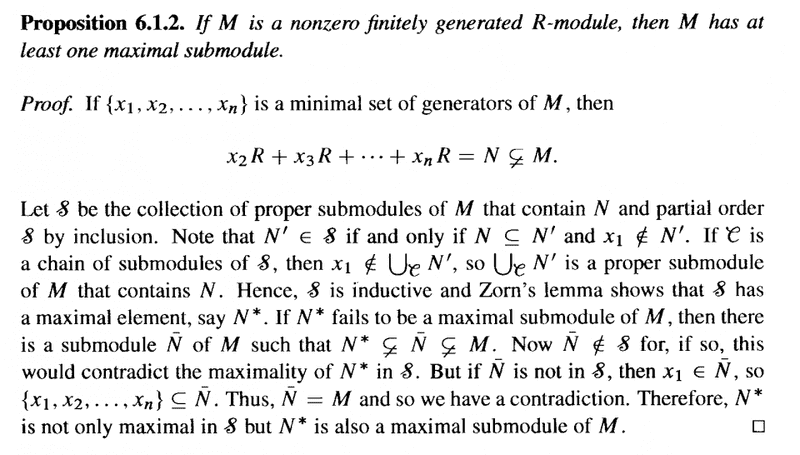
Now ... in the above proof of Proposition 6.1.2, Bland writes the following:"... ... If $\mathscr{C}$ is a chain of submodules of $\mathscr{S}$, then $x_1 \notin \bigcup_\mathscr{C}$ , so $\bigcup_\mathscr{C}$ is a proper submodule of $M$ and contains $N$. Hence $\mathscr{S}$ is inductive ... ...My question is as follows: Why does Bland bother to show that $\bigcup_\mathscr{C}$ is a proper submodule of $M$ that contains $N$ ... presumably he is showing that any chain of submodules in $\mathscr{S}$ has an upper bound ... is that right?
... ... but why does he need to do this as the largest submodule in the chain would be an upper bound ... ... ?Hope someone can help ... ...

PeterNOTE: My apologies for not being able to exactly reproduce Bland's embellished S in the above text ...

 

[micromass]

... ... but why does he need to do this as the largest submodule in the chain would be an upper bound ... ... ?

Why would there be a largest one?

 

[Math Amateur]

Why would there be a largest one?

Well I was thinking of the finite case ... e.g. where for example, \mathcal{C} might be

$N'_1 \subseteq N'_2 \subseteq N'_3$

so ... $N'_3$ in this case is an upper bound on the chain $\mathcal{C}$ ... BUT ... your question me me think that my thinking does not cover the case of an infinite chain ...

In the case of an infinite chain there may be no largest submodule and so we need to have $\bigcup_\mathcal{C} N'$ as an upper bound ...Can you confirm that my thinking is now correct ...

Peter

 

[steenis]

Yes.

The upper bound of $\mathscr{C}$ need not be in $\mathscr{C}$, but is has to be in $\mathscr{S}$.