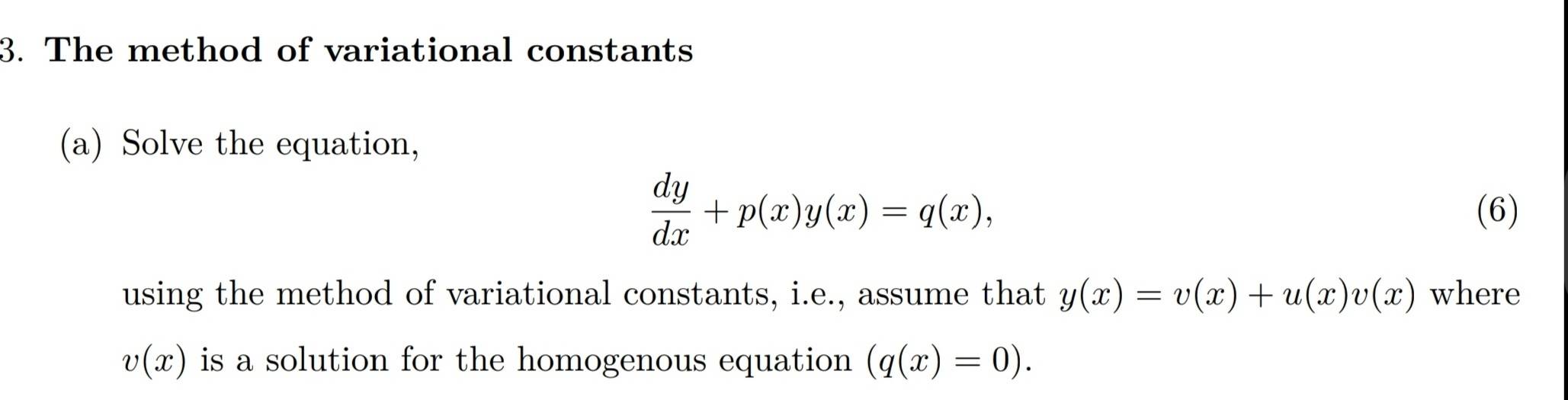
The given equation is [math]\frac{dy}{dx}+ p(x)y(x)= q(x)[/math]. The "associated homogeneous equation" is [math]\frac{dy}{dx}+ p(x)y(x)= 0[/math]. (As they say, "q(x)= 0").
That equation is "separable". [math]\frac{dy}{dx}= -p(x)y(x)[/math] and then [math]\frac{dy}{y}= -p(x)dx[/math].
Integrating both sides the general solution to the associated homogeneous equation is [math]ln(y(x))= -\int p(x)dx+ c[/math].
Take the exponential of both sides- [math]y(x)= e^{-\int p(x)dx+ c}= Ce^{-\int p(x)dx}[/math] where [math]C= e^c[/math].
Now, they are saying that you should look for a solution of the form "y(x)= v(x)+ u(x)v(x) where v(x) is a solution of the homogenous equation" (I would call this method "variation of parameters" rather than "variable parameters" also, since v(x) satisfies the homogenous equation, putting it alone into the equation will give 0 so I would use just y(x)= u(x)v(x). ) so [math]y(x)= u(x)e^{-\int p(t)dt}[/math]).
Then [math]y'= u'e^{-\int p(x)dx}- u(x)\left(p(x)e^{-\int p(x)dx}\right)= u'v- p(x)u(x)v[/math] and [math]y'+ p(x)y= u'v- p(x)u(x)v+ p(x)u(x)v= u'v= q(x)[/math]. So [math]u'= \frac{du}{dx}= \frac{q(x)}{v(x)}=[/math][math] \frac{q(x)}{e^{-\int p(t)dt}}[/math] and [math]u(x)= \int \frac{q(x)}{e^{-\int p(t)dt}}dx[/math].
For a very simple example, consider [math]y'- 2y= x^2[/math]. p(x) is the constant -2 and q(x) is [math]x^2[/math].
The associated homogeneous equation is [math]y'- 2y= 0[/math] or [math]y'= \frac{dy}{dx}= 2y[/math] which can be separated as [math]\frac{dy}{y}= 2dx[/math]. Integrating [math]ln(y)= 2x+ c[/math] and, solving for y, [math]y(x)= Ce^{2x}[/math].
Now, using "variational constants" we look for a solution to the entire equation of the form [math]y(x)= u(x)e^{2x}[/math]. Then [math]y'= u'e^{2x}+ 2ue^{2x}[/math] so the equation becomes [math]y'- 2y= u'e^{2x}+ 2ue^{2x}- 2ue^{2x}= u'e^{2x}= x^2[/tex[/math].
[math]u'= \frac{du}{dx}= x^2e^{-2x}[/math] so [math]u= \int x^2 e^{-2x}dx[/math].
To integrate that, use "integration by parts, taking [math]u= x^2[/math], so that [math]du= 2xdx[/math], and [math]dv= e^{-2x}dx[/math] so that [math]v= -\frac{1}{2}e^{-2x}[/math].
[math]\int x^2e^{-2x}dx= -\frac{1}{2}x^2e^{-2x}+ \int xe^{-2x}dx[/math].
To integrate [math]\int xe^{-2x}dx[/math] use "integration by parts" again this time with [math]u= x[/math] so that [math]du= dx[/math]and [math]dv= e^{-2x}dx[/math] so that [math]v= -\frac{1}{2}e^{-2x}[/math].
[math]\int xe^{-2x}dx= -\frac{1}{2}xe^{-2x}+ \frac{1}{2}\int e^{-2x}dx= -\frac{1}{2}xe^{-2x}- \frac{1}{4}e^{-2x}+ C[/math].