Fluid Mechanics problem - cylinder over a orifice

[Saitama]

Homework Statement

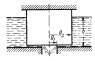
The horizontal bottom of a wide vessel with an ideal fluid has a round orifice of radius $R_1$ over which a round closed cylinder is mounted, whose radius $R_2>R_1$. The clearance between the cylinder and the bottom of vessel is very small, the fluid density is $\rho$. Find the static pressure of fluid in the clearance as a function of the distance r from the axis of the orifice (and the cylinder), if the height of the fluid is equal to $h$.

Homework Equations

The Attempt at a Solution

I think I have to apply the Bernoulli's equation but I need two points to apply the Bernoulli equation. How do I find those points?

I understand I should show some more attempt but I really can't get the intuition behind these fluid mechanics problem so I am unable to make any attempt.

Any help is appreciated. Thanks!

 

[TSny]

I think I have to apply the Bernoulli's equation but I need two points to apply the Bernoulli equation. How do I find those points?

You might start with two points where you know some information. For example, what can you learn by picking points 1 and 2 as shown?

 

[tiny-tim]

Hi Pranav-Arora!

I think I have to apply the Bernoulli's equation but I need two points to apply the Bernoulli equation.

No, you need a streamline …

Bernoulli's equation is always and only valid along a streamline.​

Once you've drawn a streamline, it should be obvious what to do next.

 

[Saitama]

Hi TSny and tiny-tim!

No, you need a streamline …

Bernoulli's equation is always and only valid along a streamline.​

Once you've drawn a streamline, it should be obvious what to do next.

I use the streamline shown by TSny and the reference line is a line passing through 2.

From Bernoulli's equation,
$$P_1+\rho gh+\frac{1}{2}\rho v_1^2=P_2+\frac{1}{2}\rho v_2^2$$

I am not sure but is $P_1=P_2$ as both points are open to atmosphere or medium outside? Also, how do I relate $v_1$ and $v_2$?

 

[tiny-tim]

Hi Pranav-Arora!

… how do I relate $v_1$ and $v_2$?

Draw circles round the hole …

since the density is constant, isn't it obvious from the geometry how the speed must increase as the circles get smaller?

 

[Saitama]

Draw circles round the hole …

since the density is constant, isn't it obvious from the geometry how the speed must increase as the circles get smaller?

Yes, I do understand that speed increases as the hole size decreases. Okay, so let the area of hole in clearance be s and that of wide vessel be S. From equation of continuity, $Sv_1=sv_2$ but what now? Do I approximate $v_1$ equal to zero?

 

[tiny-tim]

the pressure (under the cylinder) is a function of r

the pressure in the outlet should be the same as if the cylinder wasn't there

 

[Saitama]

the pressure (under the cylinder) is a function of r

the pressure in the outlet should be the same as if the cylinder wasn't there

I don't think I get this, can you please elaborate a bit more?

 

[tiny-tim]

the gauge pressure for an outlet (to the atmosphere) is zero, so that gives you v in the outlet

 

[Saitama]

the static pressure for an outlet (to the atmosphere) is zero, so that gives you v in the outlet

So that means $v_2=\sqrt{2gh}$? But the question asks something else...

 

[tiny-tim]

yes, it asks for P as a function of r, as r moves away from the rim of the hole

 

[Saitama]

yes, it asks for P as a function of r, as r moves away from the rim of the hole

Hehe tiny-tim. :P

But I don't get why you asked me to find the speed, I am honestly not sure what to do with it. How do I find the pressure as a function of r?

 

[tiny-tim]

Bernoulli's equation gives you the pressure if you know the speed.

 

[Chestermiller]

The fluid is going to be entering the hole horizontally (i.e., radially), so the volumetric throughput rate entering the hole is going to be 2\pi R_1t\sqrt{2gh}, where t is the clearance. Once you know the volumetric throughput rate, you can get the fluid velocity at any radial location r.

 

[Saitama]

The fluid is going to be entering the hole horizontally (i.e., radially), so the volumetric throughput rate entering the hole is going to be 2\pi R_1t\sqrt{2gh}, where t is the clearance. Once you know the volumetric throughput rate, you can get the fluid velocity at any radial location r.

Hi Chestermiller!

Okay, I see that you find the rate of flow of liquid but I still don't get how to find the pressure as a function of r.

Do I have to apply Bernoulli at 2 and at some distance r from the axis?

 

[Chestermiller]

Hi Chestermiller!

Okay, I see that you find the rate of flow of liquid but I still don't get how to find the pressure as a function of r.

Do I have to apply Bernoulli at 2 and at some distance r from the axis?

Yes. You have the pressure and velocity at r = R₁, and you have the velocity at arbitrary r, so you use the Bernoulli equation between these two locations to get the pressure at r.

 

[Saitama]

Yes. You have the pressure and velocity at r = R₁, and you have the velocity at arbitrary r, so you use the Bernoulli equation between these two locations to get the pressure at r.

Thanks Chestermiller and tiny-tim!

Applying Bernoulli at 2 and at a distance r,
$$P_o+\frac{1}{2}\rho(\sqrt{2gh})^2=P(r)+\frac{1}{2}\rho v^2$$
From equation of continuity, I have
$$A(r)v=2\pi R_1t\sqrt{2gh} \Rightarrow 2\pi rtv=2\pi R_1t\sqrt{2gh} \Rightarrow v=\frac{R_1\sqrt{2gh}}{r}$$

Substituting in Bernoulli and solving for P(r),
$$\boxed{P(r)=P_o+\rho gh\left(1-\frac{R_1^2}{r^2}\right)}$$
($P_o$ is the atmospheric pressure)

 

[tiny-tim]

yes, that looks right

 

[Saitama]

yes, that looks right

Thanks tiny-tim! :)

I compared my answer with the answer given in book. The book shows the condition that $R_1<r<R_2$. I don't get this. Why not $R_1 \leq r \leq R_2$? The P(r) I have found is valid for $R_1$, I am not sure about $R_2$ though.

 

[tiny-tim]

The P(r) I have found is valid for $R_1$, I am not sure about $R_2$ though.

i agree with you …

it seems obviously valid for R₁, but "at" (or even near) R₂ there's likely to be some "edge effect" (related to the movement not being entirely horizontal)

all this is academic … for an "ideal" fluid and "ideal" cylinder, your limits are just as good as the book's! ​

 

[Saitama]

i agree with you …

it seems obviously valid for R₁, but "at" (or even near) R₂ there's likely to be some "edge effect" (related to the movement not being entirely horizontal)

all this is academic … for an "ideal" fluid and "ideal" cylinder, your limits are just as good as the book's! ​

Thank you once again tiny-tim!