Guillem_dlc
- 188
- 17
- Homework Statement
- A two-dimensional jet of ##10\, \textrm{cm}## height sprays water at ##10\, \textrm{m}/\textrm{s}## which impacts, without friction, on an inclined plate...
DATA: ##\rho =1000\, \textrm{kg}/\textrm{m}^3##, ##c_1=10\, \textrm{m}/\textrm{s}##, ##h_1=10\, \textrm{cm}=0,10\, \textrm{m}##
Working hypothesis
- Stationary regime
- Uniform properties
- Incompressible flow
- Relevant Equations
- Continuity equation, Bernoulli equation
I was looking at an example of fluid mechanics and I don't understand this.
Statement figures:
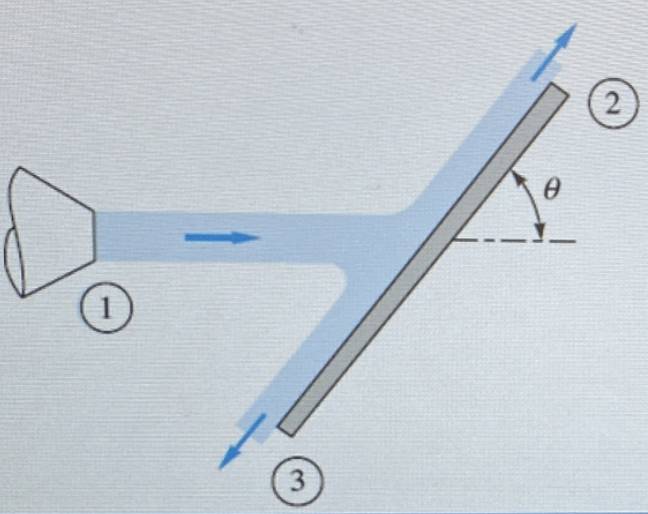

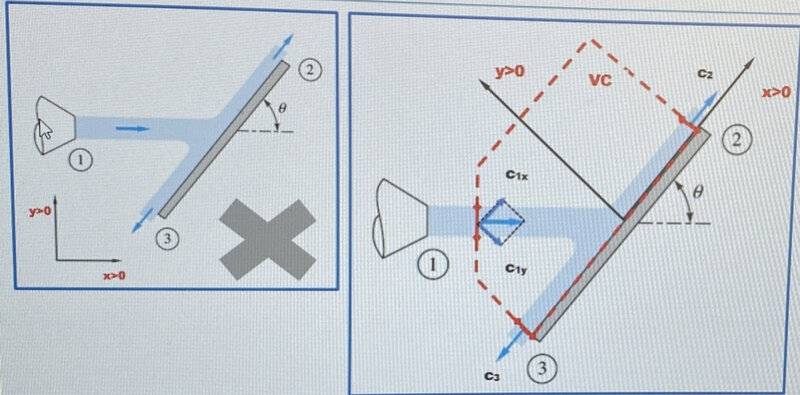
CONTINUITY EQUATION
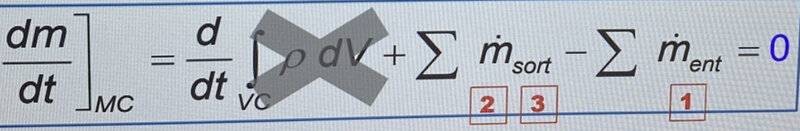
$$\left. \dfrac{dm}{dt}\right]_{MC}=(\dot{m}_2+\dot{m}_3)-\dot{m}_1=0$$
$$\dot{m}_1=\dot{m}_2+\dot{m}_3$$
$$\rho c_1A_1=\rho c_2A_2+\rho c_3A_3$$
$$\rho c_1 h1=\rho c_2 a1+\rho c_3 b1 \rightarrow \textrm{unit width}$$
$$\boxed{c_1h=c_2a+c_3b}$$
GENERALISED BERNOULLI EQUATION WITHOUT MACHINE
1-2
$$\dfrac{p_2-p_1}{\rho}+\dfrac{c_2^2-c_1^2}{2}+g(z_2-z_1)+w_{\textrm{roz}}=0 \,\,\, \left[ \dfrac{\textrm{J}}{\textrm{kg}}\right] \left\{ \begin{array}{l}
p_1=p_2=p_{\textrm{atm}}=0 \\
z_1=z_2=0 \\
w_{\textrm{roz}}=0
\end{array}\right.$$
1-3
$$\dfrac{c_2^2-c_1^2}{2}=0\rightarrow c_1=c_2$$
$$\dfrac{p_3-p_1}{\rho}+\dfrac{c_3^2-c_1^2}{2}+g(z_3-z_1)+w_{\textrm{roz}}=0\,\,\, \left[ \dfrac{\textrm{J}}{\textrm{kg}}\right] \left\{
\begin{array}{l}
p_1=p_3=p_{\textrm{atm}}=0 \\
z_1=z_3=0 \\
w_{\textrm{roz}}=0
\end{array}\right.$$
$$\dfrac{c_3^2-c_1^2}{2}=0\rightarrow c_1=c_3 \quad \boxed{c_1=c_2=c_3=c}$$
Then,
$$c_1h=c_2a+c_3b$$
$$c_1=c_2=c_3=c$$
$$\boxed{h=a+b}$$
Equation Quantity Movement 1-2
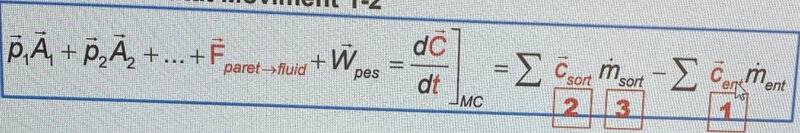
$$\overrightarrow{F}_{\textrm{paret}\rightarrow \textrm{fluid}}=(\overrightarrow{c}_2\dot{m}_2+\overrightarrow{c}_3\dot{m}_3)-\overrightarrow{c}_1\dot{m}_1$$
x-axis
$$F_{p\rightarrow f(x)}=c_{2x}\rho c_{2x}A_2+(-)c_{3x}\rho c_{3x}A_3-c_{1x}\rho c_{1x}A_1$$
$$F_{p\rightarrow f(x)}=c^2\rho 1[a-b\cos (\alpha)h]=\tau A=0\rightarrow \textrm{Frictionless}$$
$$\cos (\alpha)h=a-b$$
y-axis
$$F_{p\rightarrow f(y)}=c_{2y}\rho c_{2y}A_2+c_{3y}\rho c_{3y}A_3-(-)c_{1y}\rho c_{1y}A_1$$
$$F_{p\rightarrow f(y)}=c^2\rho h\sin (\alpha)$$
Then,
$$\left\{ \begin{array}{l}
h = a+b\\
\cos (\alpha)h=a-b
\end{array}
\right.$$
$$\alpha :=\dfrac{45\pi}{180}=0.785$$
$$h:=0.1$$
We solve the system for ##a## and ##b##:
$$(0.08536,0.01464)$$
$$\rho := 1000$$
$$c1:=10$$
$$Fpfz:=\rho \cdot c1^2\cdot h\cdot \sin (\alpha)=7071\, \textrm{N}$$
What I don't understand is the following (which has appeared before):
x-axis:
$$\tau A=0\rightarrow \textrm{Frictionless}$$
$$\cos (\alpha)h=a-b$$
$$\alpha :=\dfrac{45\pi}{180}=0.785$$
So the ##h## would be about the size of the 1, wouldn't it?
Statement figures:
CONTINUITY EQUATION
$$\left. \dfrac{dm}{dt}\right]_{MC}=(\dot{m}_2+\dot{m}_3)-\dot{m}_1=0$$
$$\dot{m}_1=\dot{m}_2+\dot{m}_3$$
$$\rho c_1A_1=\rho c_2A_2+\rho c_3A_3$$
$$\rho c_1 h1=\rho c_2 a1+\rho c_3 b1 \rightarrow \textrm{unit width}$$
$$\boxed{c_1h=c_2a+c_3b}$$
GENERALISED BERNOULLI EQUATION WITHOUT MACHINE
1-2
$$\dfrac{p_2-p_1}{\rho}+\dfrac{c_2^2-c_1^2}{2}+g(z_2-z_1)+w_{\textrm{roz}}=0 \,\,\, \left[ \dfrac{\textrm{J}}{\textrm{kg}}\right] \left\{ \begin{array}{l}
p_1=p_2=p_{\textrm{atm}}=0 \\
z_1=z_2=0 \\
w_{\textrm{roz}}=0
\end{array}\right.$$
1-3
$$\dfrac{c_2^2-c_1^2}{2}=0\rightarrow c_1=c_2$$
$$\dfrac{p_3-p_1}{\rho}+\dfrac{c_3^2-c_1^2}{2}+g(z_3-z_1)+w_{\textrm{roz}}=0\,\,\, \left[ \dfrac{\textrm{J}}{\textrm{kg}}\right] \left\{
\begin{array}{l}
p_1=p_3=p_{\textrm{atm}}=0 \\
z_1=z_3=0 \\
w_{\textrm{roz}}=0
\end{array}\right.$$
$$\dfrac{c_3^2-c_1^2}{2}=0\rightarrow c_1=c_3 \quad \boxed{c_1=c_2=c_3=c}$$
Then,
$$c_1h=c_2a+c_3b$$
$$c_1=c_2=c_3=c$$
$$\boxed{h=a+b}$$
Equation Quantity Movement 1-2
$$\overrightarrow{F}_{\textrm{paret}\rightarrow \textrm{fluid}}=(\overrightarrow{c}_2\dot{m}_2+\overrightarrow{c}_3\dot{m}_3)-\overrightarrow{c}_1\dot{m}_1$$
x-axis
$$F_{p\rightarrow f(x)}=c_{2x}\rho c_{2x}A_2+(-)c_{3x}\rho c_{3x}A_3-c_{1x}\rho c_{1x}A_1$$
$$F_{p\rightarrow f(x)}=c^2\rho 1[a-b\cos (\alpha)h]=\tau A=0\rightarrow \textrm{Frictionless}$$
$$\cos (\alpha)h=a-b$$
y-axis
$$F_{p\rightarrow f(y)}=c_{2y}\rho c_{2y}A_2+c_{3y}\rho c_{3y}A_3-(-)c_{1y}\rho c_{1y}A_1$$
$$F_{p\rightarrow f(y)}=c^2\rho h\sin (\alpha)$$
Then,
$$\left\{ \begin{array}{l}
h = a+b\\
\cos (\alpha)h=a-b
\end{array}
\right.$$
$$\alpha :=\dfrac{45\pi}{180}=0.785$$
$$h:=0.1$$
We solve the system for ##a## and ##b##:
$$(0.08536,0.01464)$$
$$\rho := 1000$$
$$c1:=10$$
$$Fpfz:=\rho \cdot c1^2\cdot h\cdot \sin (\alpha)=7071\, \textrm{N}$$
What I don't understand is the following (which has appeared before):
x-axis:
$$\tau A=0\rightarrow \textrm{Frictionless}$$
$$\cos (\alpha)h=a-b$$
$$\alpha :=\dfrac{45\pi}{180}=0.785$$
So the ##h## would be about the size of the 1, wouldn't it?