Following an example. Implies L{2du/dt+1}=2s+1. I'm Confused.

[james_a]

So I am reading a handout on transfer functions, and I got to this one example that doesn't seem right to me - which usually means I'm missing something.

It looks like this:

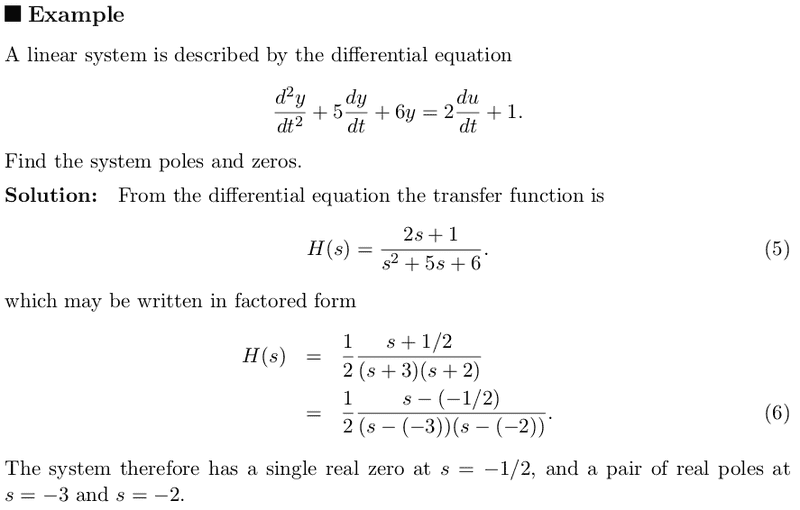
My understanding is that the numerator in H(s) is supposed to be the laplace transform of the input for the differential equation, so N(s)=L{2du/dt+1}.

I'm not sure how the author got {2du/dt+1}=2s+1. Isn't L{1} supposed to be 1/s? And I'm not sure what to make of L{2du/dt}.

Maybe the author is using "u" to notate the unit step function, so du/dt would be the dirac delta function δ(t), and L{2δ(t)}=2 . Still, where does 2s come from? Why wouldn't they have just written 2δ(t) in the first place? And that still doesn't explain how, apparently, according to the author L{1}=1.

Any clarification is greatly appreciated.

 

[FactChecker]

A derivative in the t-space gives multiplication by s in the s-space. And integration in the t-space gives division by s in the s-space.

(For proofs, see

and