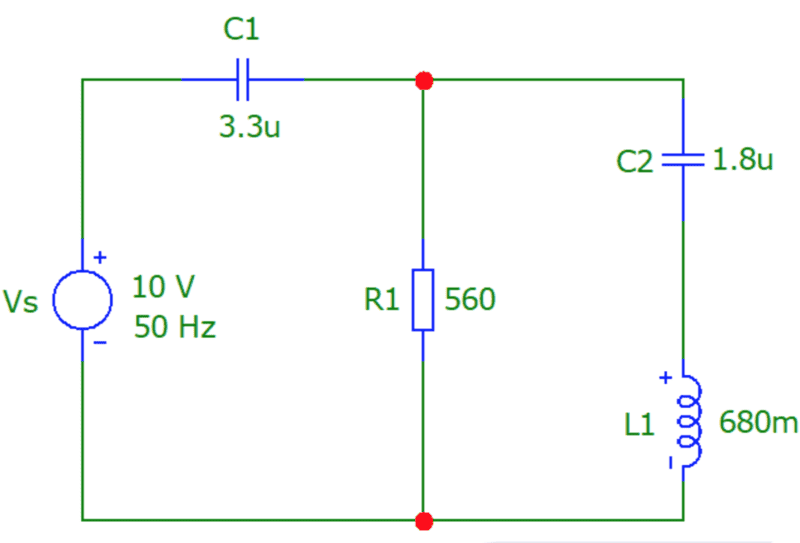
I just wanted to share this because I think some posters and future readers might find this of interest even though I think the question has already been answered very well.
I personally think for a graphing problem, that this circuit is intuitive even for an introductory circuit course. That's because introductory courses cover voltage dividers, and that series capacitance (C) and shunt resistance (R) behaves like a high-pass filter (HPF). In the introductory course I took they covered resonance circuits such as series inductance (L) and capacitance, and also all parallel L and C. Series L and C behaves like a thru short circuit at resonance.
So when I look at this circuit I'm seeing here looks like a HPF, then I see there series L and C that would behave like a short to ground at resonance. The plots I saw above behave very close to my expectation, which can be done by eyeball inspection using this way of thinking.
Something that was not covered in my introductory course... I learned it at my first full-time position and someone recommended a book that covered it really well (Fundamentals of Power Electronics by Erickson)... it's call the Middlebrook Extra Element Theorem. I think this is a very good use case for that one. Again: I look at it and say I know how some of the circuit behaves without some elements. I can remove that L and C that is in series to ground and say "I know how this HPF behaves, but what does this extra element(s) do?" Check it out. I recommend trying it out with a well-known resistive voltage divider to see if you can apply it, and then repeat the procedures on this circuit here.
https://en.wikipedia.org/wiki/Extra_element_theorem
Below I just did it with the resistive voltage divider because we know 2R parallel to 2R behaves like R, and we know how a voltage divider behaves when the resistors are the same. It's obviously not required for this problem, but I do think it's interesting and I come across a lot of cases where I understand really well how a circuit behaves if it didn't have some darn extra element :) now the problem is no more, and it's just fun to explore a bunch of different circuits or trying to break some of them. I use it for work, and even in classes where I'll stumble upon in an exam the professor is trying to be tricky and turned a known good circuit into something that's a little off.
Something to be cautious of is that I did not always understand at the beginning was the nulling. You should follow the procedures of placing a test source there and getting the voltage and current that nulls Vout (drives it to zero). In this particular case: It can be done by inspection because Znull happens to be a short anyways, but directly shorting to ground or making Vout zero is not the right approach, and it just so happens that you can "get away with it" (or get lucky) in this simple case but if you tried it where the extra element was parallel to the series R, then you might not be so lucky.