Frequency selective surface radome

[garryA]

TL;DR Summary

Transmission coefficient vs Reflection coefficient of FSS radome

So I was reading about frequency selective surface radome, basically this is the kind of cover over the antenna which allow a certain frequency wave to pass through and reflect wave at any other frequencies.

What I don't understand is the reflection vs transmission coefficient chart.
So as I understand it.
The transmission coefficient is a measure of how much of an electromagnetic wave passes through a surface
The reflection coefficient is a a measure of how much of an electromagnetic wave reflected when it hit a surface, The reflection coefficient determines the ratio of the reflected wave amplitude to the incident wave amplitude.

So logically, wouldn't the two value always opposite?
Let me give a practical example:
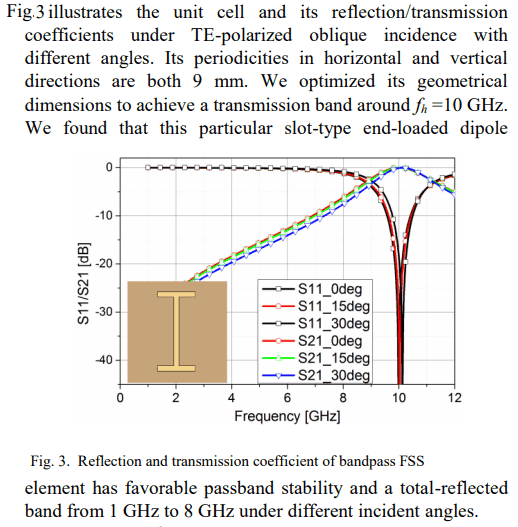
below, the band pass frequency is 10Ghz, so the transmission coefficient is 0 dB while the reflection coefficient is extremely small (like -40 dB). That make total sense because if it reflect nothing then most energy can pass through the layer

But take for example at 6Ghz, the reflection coefficient is at 0 dB, meaning it reflect everything, so how come the transmission coefficient is not at -40 dB?.
Shouldn't the shape of the two curve for reflection/transmission coefficient completely reverse each other?

 

[Baluncore]

So logically, wouldn't the two value always opposite?

Energy that is lost to heat within the material, is neither transmitted, nor reflected.

 

[garryA]

Energy that is lost to heat within the material, is neither transmitted, nor reflected.

I got your point, but to be able to be converted to heat, the wave must entered the material first, so the fraction of energy that get reflected can’t be transmitted or converted to heat.
Let me clarify my point with an example:
Let say the amount of energy of the wave at the start is 100%. Once it hit the radome, 99.99% of that get reflected. So I would expected that the amount that can be transmitted is at most 0.001%. But the chart basically telling me that 99.99% energy get reflected but 10% get transmitted. That why for me it make no sense

 

[tech99]

If the radome is loss free, the transmitted power and the reflected power should always add up to incident power. To compare transmitted power and reflected power you have to deduct the two powers. To do this you cannot use decibels, you must first convert from decibels (relative to incident power) to watts. Then subtract the watts.
For example, incident power Pi = 1 watt, and transmitted power 1 W -1 dB = 0.8W.
So reflected power = 1 - 0.8 = 0.2W. Or if preferred, 0.2W = -7dB.

 

[garryA]

If the radome is loss free, the transmitted power and the reflected power should always add up to incident power.

Yes, that is my deduction as well

To compare transmitted power and reflected power you have to deduct the two powers. To do this you cannot use decibels, you must first convert from decibels (relative to incident power) to watts. Then subtract the watts.
For example, incident power Pi = 1 watt, and transmitted power 1 W -1 dB = 0.8W.
So reflected power = 1 - 0.8 = 0.2W. Or if preferred, 0.2W = -7dB. if I understand correctly,

the incident power will be reflected before it can be transmitted
So for example in the point in the graph bellow
let say the incident power is 100W, then the reflected power is also 100W
how come the transmitted power is still there?
Shouldn't the shape of two line completely opposite?

 

[renormalize]

let say the incident power is 100W, then the reflected power is also 100W

No, roughly 5W is transmitted through the radome, leaving a reflected power of about 95W by conservation of energy. That's an S11 of -0.22dB, only slightly below zero and nearly impossible to see on the dB graph. Observe also that the S11 and S21 lines cross at a value of -3dB, corresponding to 50% transmitted/50% reflected powers, which demonstrates that no power loss due to absorption is included in these model results.

 

[tech99]

The graph is plotted inm decibels. If it was plotted in watts, then the two plots would be the inverse of each other.

 

[garryA]

No, roughly 5W is transmitted through the radome, leaving a reflected power of about 95W by conservation of energy. That's an S11 of -0.22dB, only slightly below zero and nearly impossible to see on the dB graph. Observe also that the S11 and S21 lines cross at a value of -3dB, corresponding to 50% transmitted/50% reflected powers, which demonstrates that no power loss due to absorption is included in these model results.
View attachment 323599

Thank you, it make sense to me now.
Also If i understand correctly , if we put a radar behind this radome, then the process of reflected happen twice right? one when the wave go out the randome, and one when it come back