- #1
Math Amateur
Gold Member
MHB
- 3,998
- 48
I am reading the book, Basic Abstract Algebra by P.B. Bhattacharya, S.K. Jain, and S.R. Nagpaul ... ... and am currently focused on Chapter 2: Integers, Real Numbers and Complex Numbers ...I need help with an aspect of the proof of the Fundamental Theorem of Arithmetic in Section 1.3 ... ...The Fundamental Theorem of Arithmetic and its proof as presented by Bhattacharya et al reads as follows:

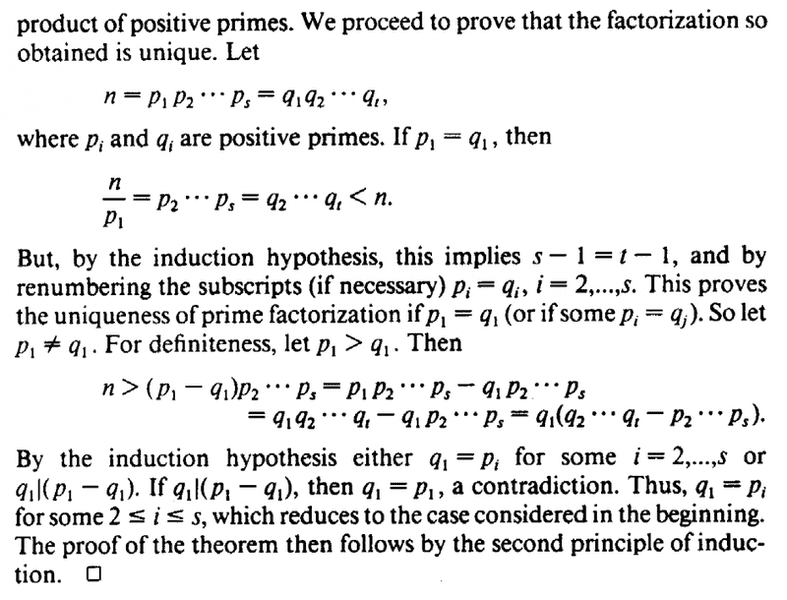
In the above text we read the following:" ... ... So let ##p_1 \neq q_1##. For definiteness, let ##p_1 \gt q_1##. Then
##n \gt (p_1 - q_1) p_2 \ ... \ p_s = p_1p_2 \ ... \ p_s - q_1p_2 \ ... \ p_s##
##= q_1q_2 \ ... \ q_t - q_1p_2 \ ... \ p_s = q_1 (q_2 \ ... \ q_t - p_2 \ ... \ p_s)##
By the induction hypothesis either ##q_1 = p_i## for some ##i = 2, \ ... \ , s## or
##q_1 | (p_1 - q_1)##. ... ... "I do not follow how
##n \gt (p_1 - q_1) p_2 \ ... \ p_s = p_1p_2 \ ... \ p_s - q_1p_2 \ ... \ p_s##
##= q_1q_2 \ ... \ q_t - q_1p_2 \ ... \ p_s = q_1 (q_2 \ ... \ q_t - p_2 \ ... \ p_s)##
leads to
either ##q_1 = p_i## for some ##i = 2, \ ... \ , s## or ##q_1 | (p_1 - q_1)##. ... ...
Can someone slowly and clearly explain exactly how the above follows ...Hope someone can help ... ...
Peter
======================================================The above refers to what Bhattacharya et al call the Second Principle of Induction ... this principle reads as follows in their text ... ... :
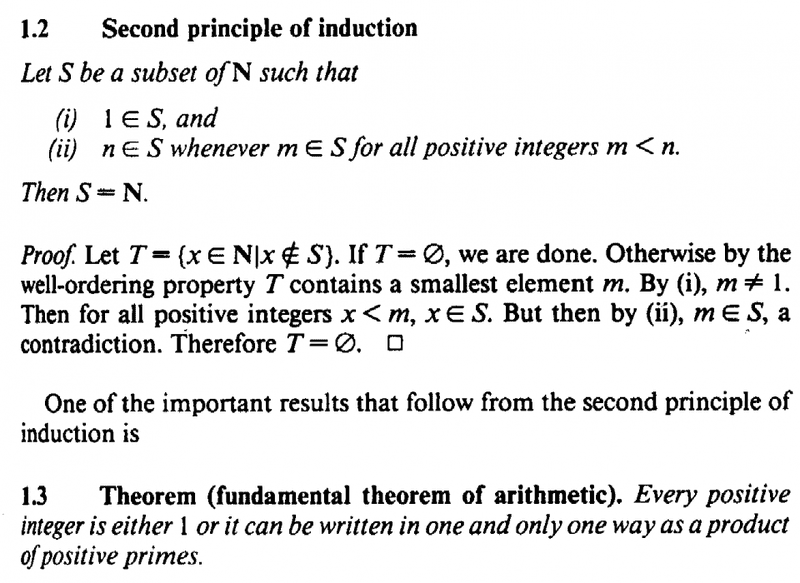
In the above text we read the following:" ... ... So let ##p_1 \neq q_1##. For definiteness, let ##p_1 \gt q_1##. Then
##n \gt (p_1 - q_1) p_2 \ ... \ p_s = p_1p_2 \ ... \ p_s - q_1p_2 \ ... \ p_s##
##= q_1q_2 \ ... \ q_t - q_1p_2 \ ... \ p_s = q_1 (q_2 \ ... \ q_t - p_2 \ ... \ p_s)##
By the induction hypothesis either ##q_1 = p_i## for some ##i = 2, \ ... \ , s## or
##q_1 | (p_1 - q_1)##. ... ... "I do not follow how
##n \gt (p_1 - q_1) p_2 \ ... \ p_s = p_1p_2 \ ... \ p_s - q_1p_2 \ ... \ p_s##
##= q_1q_2 \ ... \ q_t - q_1p_2 \ ... \ p_s = q_1 (q_2 \ ... \ q_t - p_2 \ ... \ p_s)##
leads to
either ##q_1 = p_i## for some ##i = 2, \ ... \ , s## or ##q_1 | (p_1 - q_1)##. ... ...
Can someone slowly and clearly explain exactly how the above follows ...Hope someone can help ... ...
Peter
======================================================The above refers to what Bhattacharya et al call the Second Principle of Induction ... this principle reads as follows in their text ... ... :

