Gauss' law in differential form

[Leo Liu]

Homework Statement

.

Relevant Equations

.

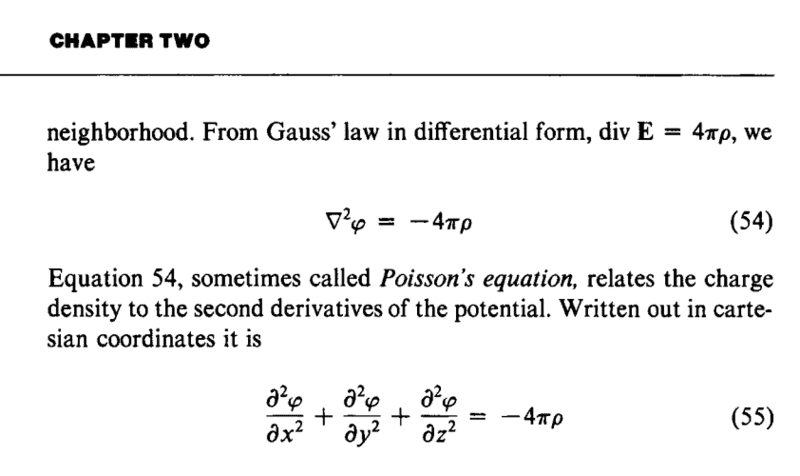
My book claims that the diff. form of Gauss' law is
$$\nabla\cdot\mathbf E=4\pi\rho$$
Can someone tell me why it isn't $\nabla\cdot\mathbf E=\rho/\epsilon_0$?

 

[TSny]

It's the system of units being used.

 

[hutchphd]

I'm sure @TSny would go into more detail but trust me you do not wish to do that and he has chosen not to. Just get used to different factors of 4pi and epsilon and mu in equations and understand there is no problem. The pictures in your head should not depend upon these details.

 

[Leo Liu]

I'm sure @TSny would go into more detail but trust me you do not wish to do that and he has chosen not to. Just get used to different factors of 4pi and epsilon and mu in equations and understand there is no problem. The pictures in your head should not depend upon these details.

Thanks. But why do we need two sets of units for EM? Doesn't SI suffice all of our needs?

 

[hutchphd]

History and politics

 

[Orodruin]

Thanks. But why do we need two sets of units for EM? Doesn't SI suffice all of our needs?

You could use SI for everything. It is not always handy though.

 

[rude man]

Both systems have their pluses and minuses.
Conventionally, theoretical physics, especially particle physicists, tend to go with cgs. But today there seems to be a strong trend to go rationalized mks, aka SI. Personally I won't deal with cgs though that is what I had to deal with in my own introductory physics course. A long time ago, thank goodness.

One thing I don't like about cgs is that it has no separate unit for charge Q. For an EE like myself that is unacceptable. I'll let the particle physicists defend cgs.

Of course, the presence/absence of Q is a tradeoff of sorts. In general, increasing the number of characters in a vocabulary shortens the text but at the expense of extra characters in the "alphabet". Cf. English vs. Chinese.

Another example is avoidance of extra parameters even within a given system. Some teachers prefer a minimum of parameters, others like abbreviated text. E.g. you can avoid $\bf D$ and $\bf H$ since $\bf D = \epsilon \bf E$ and $\bf B = \mu \bf H$ but personally I find that awkward. Clutters the Maxwell equations, for example. Richard Feynman even avoids using $\mu$, sticking to $c$ and $\epsilon$. If you're SI it makes his cgs-based Lectures hard to follow at times.

Etc.