Generalisation of terms in a series

[jackiepollock]

Homework Statement

I'm stuck at understanding the generalisation of the terms in a series

Relevant Equations

Maclaurin series

Hello. I'm not sure how the generalisation comes about (where I circle).

I assume that r means the the rth derivative of f(x). If that's the case, as I plug 3 = r into this generalisation, the third derivative term should equal to (-1)^3x^7 /7!, but the third derivative term is -1x^3/3!.

What's the problem?

 

[Delta2]

$\frac{(-1)^r}{(2r+1)!}x^{2r+1}$ is the $(2r+1)$ term of the series, which means that for r=3 you get the $2r+1=2\cdot 3+1=7$ that is the 7th term of the series. The 3rd term of the series is for the $r'$ such that $2r'+1=3$ or $r'=1$.
In case you wonder what happens to the $2r$ terms of the series, they are all zero because the corresponding derivative at 0 $f^{(2r)}(0)=0$ is equal to zero

Essentially what I am telling you is that the even terms of the series are all zeros and the odd terms are $\frac{(-1)^r}{(2r+1)!}x^{2r+1}$

 

[vela]

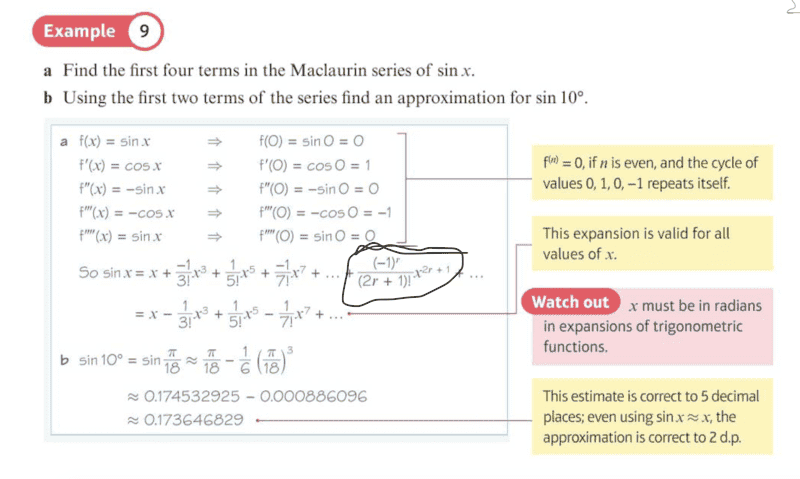
I assume that r means the the rth derivative of f(x). If that's the case, as I plug 3 = r into this generalisation, the third derivative term should equal to (-1)^3x^7 /7!, but the third derivative term is -1x^3/3!.

What's the problem?

Your assumption is incorrect. The $r$ in the expansion for $\sin x$ and the $r$ in the general formula for the Maclaurin series are different variables. Note in the general formula, the exponent of $x$ is equal to the order of the derivative, so the $x^7$ term is matched to the seventh derivative of $f$.

 

[Delta2]

Your assumption is incorrect. The $r$ in the expansion for $\sin x$ and the $r$ in the general formula for the Maclaurin series are different variables. Note in the general formula, the exponent of $x$ is equal to the order of the derivative, so the $x^7$ term is matched to the seventh derivative of $f$.

Yes I think what confuses him is that the even terms of the series are zero for the specific expansion of $\sin x$ and that they use the same letter r for the generic series formula and for the specific series of sinx.