- #1
Bashyboy
- 1,421
- 5
Homework Statement
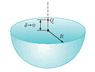
A particle with charge Q is located immediately above the center of the flat face of a hemisphere of radius R as shown in the figure below.
(a) What is the electric flux through the curved surface? (Use any variable or symbol stated above along with the following as necessary: ε0.)
(b) What is the electric flux through the flat face? (Use any variable or symbol stated above along with the following as necessary: ε0.)
Homework Equations
The Attempt at a Solution
Taking the surface area equation for a sphere, and dividing by two: [itex]2 \pi r^2[/itex]
Area equation for a circle: [itex]\pi r^2[/itex]
The expression for (a): [itex]\Phi = E2 \pi r^2[/itex]
The expression for (b): [itex]\Phi = E \pi r^2[/itex]
These are incorrect. What did I do wrong?
Attachments
Last edited: