MatinSAR
- 673
- 204
- Homework Statement
- Find the geodesic on a sphere and on a plane in 2D.
- Relevant Equations
- Calculus of variation.
I start with the 2D plane. Suppose y(x) is the curve that connects these two points. Its length is given by:
$$S=\int_1^2 \, ds=\int_1^2 (1+y'^2)^{\frac {1}{2}} \, dx$$ Applying Euler's equation we get:$$\frac {\partial f} {\partial y'}=A$$$$\dfrac {y'}{(1+y'^2)^{\frac {1}{2}}}=A$$ $$y'^{2}=\dfrac {A^2}{1-A^2}$$$$\dfrac {dy}{dx}=\pm (\dfrac {A^2}{1-A^2})^{\frac 1 2}=B$$$$dy=Bdx$$$$y=Bx+C$$
So the shortest distance is a line that connects the points. I know it is one of the easiest questions in calculus of variation, I just wanted to know If I learnt it correctly. Any suggustion would be appreciated.
For the sphere, My answer is completely similar to this:
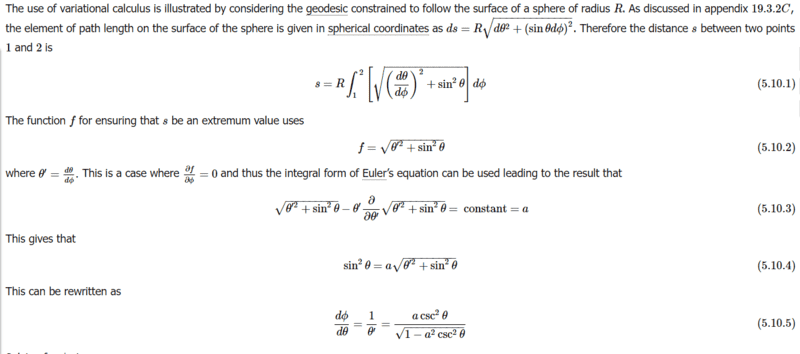
My problem is that I don't know how to obtain 5.10.5 using 5.10.4 ...
Many thanks.
$$S=\int_1^2 \, ds=\int_1^2 (1+y'^2)^{\frac {1}{2}} \, dx$$ Applying Euler's equation we get:$$\frac {\partial f} {\partial y'}=A$$$$\dfrac {y'}{(1+y'^2)^{\frac {1}{2}}}=A$$ $$y'^{2}=\dfrac {A^2}{1-A^2}$$$$\dfrac {dy}{dx}=\pm (\dfrac {A^2}{1-A^2})^{\frac 1 2}=B$$$$dy=Bdx$$$$y=Bx+C$$
So the shortest distance is a line that connects the points. I know it is one of the easiest questions in calculus of variation, I just wanted to know If I learnt it correctly. Any suggustion would be appreciated.
For the sphere, My answer is completely similar to this:
My problem is that I don't know how to obtain 5.10.5 using 5.10.4 ...
Many thanks.
