- #1
Math Amateur
Gold Member
MHB
- 3,998
- 48
I am reading Andrew McInerney's book: First Steps in Differential Geometry: Riemannian, Contact, Symplectic ...
I am currently focussed on Chapter 3: Advanced Calculus ... and in particular I am studying Section 3.3 Geometric Sets and Subspaces of [itex]T_p ( \mathbb{R}^n )[/itex] ...
I need help with a basic aspect of Example 3.3.7 ...
Example 3.3.7 reads as follows:
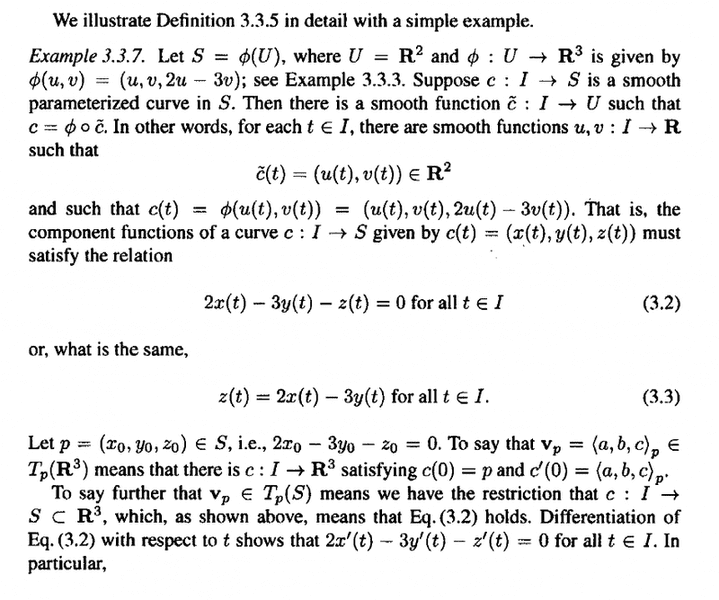
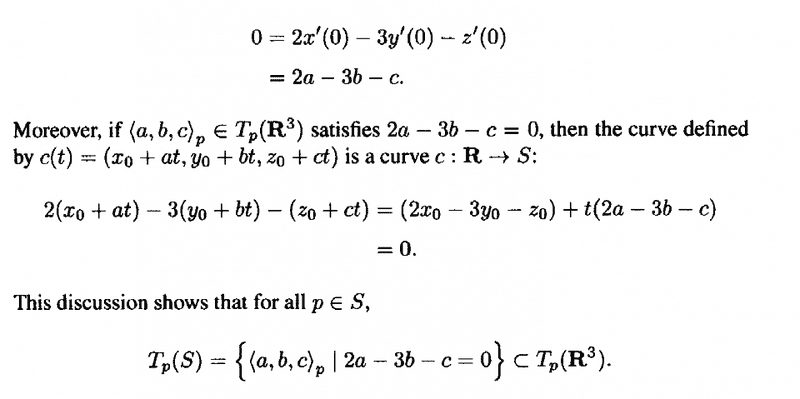
Question 1
In the above text we read:
" ... ... Let [itex]p = ( x_0, y_0, z_0 ) \in S[/itex] i.e. [itex]2 x_0 - 3 y_0 - z_0 = 0[/itex]. ... ... "... BUT ... as I read the example ... ... we have that [itex]2 x_0 - 3 y_0 - z_0 = 0[/itex] is the equation of [itex]c(t)[/itex] at [itex]( x_0, y_0, z_0 )[/itex] ... AND ... again as I see it ... this is not all of [itex]S[/itex] as [itex]c[/itex] maps [itex]I[/itex] into [itex]S[/itex] ... thus a general point [itex]p = ( x_0, y_0, z_0 ) \in S[/itex] may not satisfy the equation as it may not be in the range of [itex]c[/itex] ...Can some please clarify my issue with the example... ? (I hope I have made my question clear ..)===========================================================
*** EDIT ***
After some reflection I now feel that all points in [itex]S = \phi (U) = ( u, v, 2u - 3v )[/itex] satisfy the equation [itex]2x - 3y - z = 0[/itex] ... so a particular point [itex]p = ( x_0, y_0, z_0)[/itex] obviously satisfies [itex]2x_0 - 3y_0 - z_0 = 0[/itex] ... is that right ... ?
Please let me know if my edit is correct ...
==========================================================
Question 2
In the above text we read:
" ... ... This discussion shows that for all [itex]p \in S[/itex],
[itex]T_p (S) = \{ (a, b, c)_p \ \ | \ \ 2a - 3b - c = 0 \} \subset T_p ( \mathbb{R}^3 ) [/itex]
... ... ... ... "Now, it seems that vectors at [itex]p = (x_0, y_0, z_0)[/itex] that have components [itex]a, b, c[/itex] respectively which obey the equation, [itex]2a - 3b - c =0[/itex] are (I think?) in [itex]S[/itex] ... ... so this would mean that [itex]T_p(S)[/itex] is a subset of [itex]S[/itex] ... Is that correct ...?Question 3
Does anyone know of any books with a simple approach to tangent spaces replete with a number of worked/computational exercises ...?
Hope someone can help with the above questions ...
PeterI have made a simple diagram of my understanding of the mappings involved ... as follows ... ...
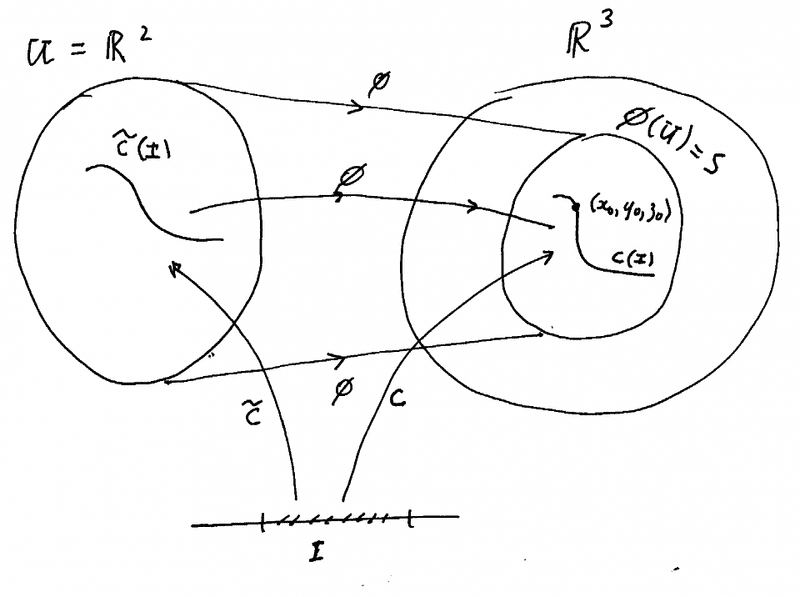 Is the above diagram a correct representation of the mappings involved?
Is the above diagram a correct representation of the mappings involved?
To help to give some of the context and some explanation of the theory and notation relevant to the above I am providing McInerney's introduction to Section 3.3 as follows:

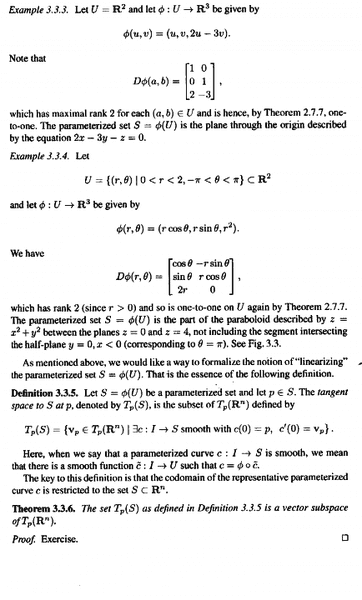
I am currently focussed on Chapter 3: Advanced Calculus ... and in particular I am studying Section 3.3 Geometric Sets and Subspaces of [itex]T_p ( \mathbb{R}^n )[/itex] ...
I need help with a basic aspect of Example 3.3.7 ...
Example 3.3.7 reads as follows:
Question 1
In the above text we read:
" ... ... Let [itex]p = ( x_0, y_0, z_0 ) \in S[/itex] i.e. [itex]2 x_0 - 3 y_0 - z_0 = 0[/itex]. ... ... "... BUT ... as I read the example ... ... we have that [itex]2 x_0 - 3 y_0 - z_0 = 0[/itex] is the equation of [itex]c(t)[/itex] at [itex]( x_0, y_0, z_0 )[/itex] ... AND ... again as I see it ... this is not all of [itex]S[/itex] as [itex]c[/itex] maps [itex]I[/itex] into [itex]S[/itex] ... thus a general point [itex]p = ( x_0, y_0, z_0 ) \in S[/itex] may not satisfy the equation as it may not be in the range of [itex]c[/itex] ...Can some please clarify my issue with the example... ? (I hope I have made my question clear ..)===========================================================
*** EDIT ***
After some reflection I now feel that all points in [itex]S = \phi (U) = ( u, v, 2u - 3v )[/itex] satisfy the equation [itex]2x - 3y - z = 0[/itex] ... so a particular point [itex]p = ( x_0, y_0, z_0)[/itex] obviously satisfies [itex]2x_0 - 3y_0 - z_0 = 0[/itex] ... is that right ... ?
Please let me know if my edit is correct ...
==========================================================
Question 2
In the above text we read:
" ... ... This discussion shows that for all [itex]p \in S[/itex],
[itex]T_p (S) = \{ (a, b, c)_p \ \ | \ \ 2a - 3b - c = 0 \} \subset T_p ( \mathbb{R}^3 ) [/itex]
... ... ... ... "Now, it seems that vectors at [itex]p = (x_0, y_0, z_0)[/itex] that have components [itex]a, b, c[/itex] respectively which obey the equation, [itex]2a - 3b - c =0[/itex] are (I think?) in [itex]S[/itex] ... ... so this would mean that [itex]T_p(S)[/itex] is a subset of [itex]S[/itex] ... Is that correct ...?Question 3
Does anyone know of any books with a simple approach to tangent spaces replete with a number of worked/computational exercises ...?
Hope someone can help with the above questions ...
PeterI have made a simple diagram of my understanding of the mappings involved ... as follows ... ...
To help to give some of the context and some explanation of the theory and notation relevant to the above I am providing McInerney's introduction to Section 3.3 as follows:
Attachments
-
 McInerney - 1 - Example 3.3.7 - Section 2.6 - PART 1 - Page 81 .png56.3 KB · Views: 810
McInerney - 1 - Example 3.3.7 - Section 2.6 - PART 1 - Page 81 .png56.3 KB · Views: 810 -
 McInerney - 2 - Example 3.3.7 - Section 2.6 - PART 2 - Page 82 .png60.2 KB · Views: 740
McInerney - 2 - Example 3.3.7 - Section 2.6 - PART 2 - Page 82 .png60.2 KB · Views: 740 -
 Peter - Figure 1 - McInerney - Example 3.3.7.png81.5 KB · Views: 717
Peter - Figure 1 - McInerney - Example 3.3.7.png81.5 KB · Views: 717 -
 McInerney - 1 - Section 3.3 - PART 1 - Page 79 .png45.5 KB · Views: 660
McInerney - 1 - Section 3.3 - PART 1 - Page 79 .png45.5 KB · Views: 660 -
 McInerney - 2 - Section 3.3 - PART 2 - Page 80 .png28.6 KB · Views: 629
McInerney - 2 - Section 3.3 - PART 2 - Page 80 .png28.6 KB · Views: 629
Last edited: