Getting the equations of motion for this SHM problem

[phantomvommand]

Summary:: I have come across a situation where I seem to get different equations of motion for an oscillating system. Please do help me find out where I went wrong.

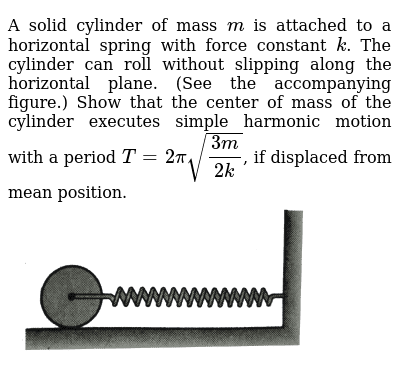
*I am not asking how to solve the problem*
I am going to consider 4 parts of the cylinder's motion, as listed below. (There is friction, denoted by f, between the cylinder and the floor.)
1. Displacement left of equilibrium, velocity towards the left
2. Displacement left, moving right
3. Displacement right, moving right
4. Displacement right, moving left

Case 1:
mx'' = -kx - f, where leftwards is taken as positive. kx is a rightwards force when displacement is leftwards, moving left implies friction is to the right.
Case 2:
mx'' = -kx + f, leftwards is positive. I have flipped the sign of friction here, because it is moving right, so friction is to the left.
Case 3:
mx'' = -kx - f, rightwards is positive.
Case 4:
mx'' = -kx + f, rightwards is positive. This is similar to Case 2, so friction is now positive too.

There are 2 different equations of motion, why are they ultimately the same?

 

[Lnewqban]

I believe that you should not be concerned with the magnitude or direction of the friction force between cylinder and surface for this problem.
The cylinder is forced to rotate, which means that its rotational inertia should be considered.

 

[haruspex]

Your approach is not going to work because the frictional force is not constant in magnitude.
It will be related to displacement. Consequently it will change sign as appropriate without needing a different sign in the equation.

 

[kuruman]

If you write Newton's second law for rotations about the point of contact P, you have a restoring torque $\tau=-kxR=I_P~\alpha$. The friction exerts no torque, for rolling without slipping $x=R\theta$ and so on.

 

[phantomvommand]

Your approach is not going to work because the frictional force is not constant in magnitude.
It will be related to displacement. Consequently it will change sign as appropriate without needing a different sign in the equation.

Thanks for this. I do realize that friction = 0.5mx’’. How do I know when to set friction to be positive, and when it is negative?

 

[haruspex]

Thanks for this. I do realize that friction = 0.5mx’’. How do I know when to set friction to be positive, and when it is negative?

You have to choose a positive direction for the frictional force. Having chosen it, that determines its sign in each of two equations: the one you quote above and the one for the horizontal F=ma balance. As long as you are consistent it will all work.
As a check, would you expect the magnitude of the acceleration to be more less than |kx/m|?

 

[hutchphd]

I do realize that friction = 0.5mx’’.

How do you know this? Because there is is a constraint as you realize. There are two equations here. One contains an unknown frictional force. The constraint equation relates this force to the motion including the sign. (As a check: The wheel rotating makes it effectively more massive when accelerated by the spring)

 

[phantomvommand]

How do you know this? Because there is is a constraint as you realize. There are two equations here. One contains an unknown frictional force. The constraint equation relates this force to the motion including the sign. (As a check: The wheel rotating makes it effectively more massive when accelerated by the spring)

I used friction*radius = I*angular acceleration.
Setting R*alpha = acceleration gives you friction as 1/2mx”.
One way to get the sign of friction is to set the anti-clockwise rotation as positive (when leftwards motion is positive). What is the constraint equation you mentioned? Thank you.

 

[hutchphd]

I used friction*radius = I*angular acceleration.

Yes that is fine. And the constraint is (formally your choice of sign) that $$r\theta =x$$ and so$$r \ddot \theta=\ddot x$$ which determines the relation chosen between x and theta