- #1
graupner1000
- 16
- 1
Hi all,
I'm hoping somebody can save me from this impending frustration induced aneurysm. The problem lies with Maple's physics package and is this:
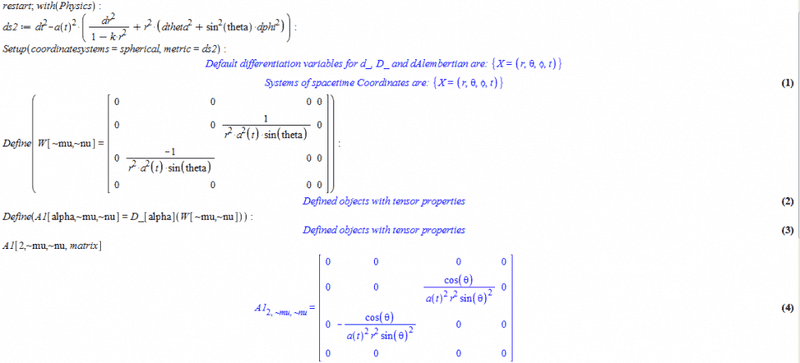
I am in an FLRW spacetime with metric
##ds^{2}=dt^{2}−a(t)^{2}(\frac{dr^{2}}{1−kr^{2}}+r^{2}d\theta^{2}+r^{2}sin^{2}(\theta)d\phi^{2})##
and a(t) is the scale factor. Now, I also have an antisymmetric, rank two tensor ##W## with components
##W^{23}=−W^{32}=\frac{1}{r^{2}a(t)^{2}sin(\theta)}##
and all others zero. So far, so straight forward. OK, next I want to take the covariant derivative of ω; rather easy with the Christoffel symbols given here http://universeinproblems.com/index...oblem_20:_Christoffel_symbols_for_FLRW_metric, and get
##\nabla_{\alpha} W^{\mu\nu} = 0##
Now, what I am doing involves many subsidiary calculations so I am doing it in Maple using the Physics package. I am confident in the answer above and have had somebody check it for me (by hand). The problem is, when telling maple to evaluate the above covariant derivative, it gives a different answer. Not only that, but seems, that giving it the same problem in three different ways results in different answers (neither of which is the one it should be). In one instance, it is the D_() command, in the second I use the "Define" command and D_() and in the other I am expanding the covariant derivative in terms of Christoffel symbols and using "Define" to input it as
d_[alpha](W[~mu,~nu]) + Christoffel[~mu,alpha,beta] W[~mu,~nu]+ Christoffel[~nu,alpha,beta] W[~mu,~nu]
The issue is less with this specific calculation, but more with whether or not I can rely on the package to give me the right answer (I'd rather not have to do it all by hand). So I basically wanted to ask where am I going wrong? Is it me or the software?
I've also attached screesshots of a minimal example . Any help would be very much appreciated. Meanwhile, I'm going to go and scream at a wall :)

I'm hoping somebody can save me from this impending frustration induced aneurysm. The problem lies with Maple's physics package and is this:
I am in an FLRW spacetime with metric
##ds^{2}=dt^{2}−a(t)^{2}(\frac{dr^{2}}{1−kr^{2}}+r^{2}d\theta^{2}+r^{2}sin^{2}(\theta)d\phi^{2})##
and a(t) is the scale factor. Now, I also have an antisymmetric, rank two tensor ##W## with components
##W^{23}=−W^{32}=\frac{1}{r^{2}a(t)^{2}sin(\theta)}##
and all others zero. So far, so straight forward. OK, next I want to take the covariant derivative of ω; rather easy with the Christoffel symbols given here http://universeinproblems.com/index...oblem_20:_Christoffel_symbols_for_FLRW_metric, and get
##\nabla_{\alpha} W^{\mu\nu} = 0##
Now, what I am doing involves many subsidiary calculations so I am doing it in Maple using the Physics package. I am confident in the answer above and have had somebody check it for me (by hand). The problem is, when telling maple to evaluate the above covariant derivative, it gives a different answer. Not only that, but seems, that giving it the same problem in three different ways results in different answers (neither of which is the one it should be). In one instance, it is the D_() command, in the second I use the "Define" command and D_() and in the other I am expanding the covariant derivative in terms of Christoffel symbols and using "Define" to input it as
d_[alpha](W[~mu,~nu]) + Christoffel[~mu,alpha,beta] W[~mu,~nu]+ Christoffel[~nu,alpha,beta] W[~mu,~nu]
The issue is less with this specific calculation, but more with whether or not I can rely on the package to give me the right answer (I'd rather not have to do it all by hand). So I basically wanted to ask where am I going wrong? Is it me or the software?
I've also attached screesshots of a minimal example . Any help would be very much appreciated. Meanwhile, I'm going to go and scream at a wall :)