- #1
DaveC426913
Gold Member
- 23,070
- 6,747
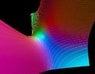
I don't have a 3D graphing program, so I'm trying to figure out the graph of y=x^z on paper.
y=x^2 forms a parabola while y=x^3 forms a ... other thing. There's got to be some sensical values to y as the exponent climbs through the values between them.
y=x^2 forms a parabola while y=x^3 forms a ... other thing. There's got to be some sensical values to y as the exponent climbs through the values between them.
Last edited: