Graphing x=t, y=1/(1+t^2), z=t^2 - Is it a Parabola?

[ineedhelpnow]

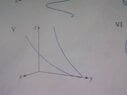
i don't know whether this goes here or under the calculator forum. i want to graph x=t y=1/(1+t^2) and z=t^2 and i keep getting a parabola. is that right because the drawing in my book is like a slanted parabola.

View attachment 3231

 

[MarkFL]

What are the ranges for $x,\,y,\,z$ as given by the parametric equations?

Also, ignoring each parametric equation in turn, what shadows would then be cast on the relevant planes?

 

[ineedhelpnow]

 

[MarkFL]

Let's ignore $z$ for the moment, and eliminate the parameter from $x$ and $y$, resulting in:

$$y=\frac{1}{1+x^2}$$

We should have a pretty good idea what this looks like...a global maximum at $y(0)=1$, a horizontal asymptote along the $x$-axis in both directions since we have an even function.

So, imagine rays of light parallel to the $z$-axis causing a shadow to be cast by the curve onto the $xy$-plane. Do we get such a curve as described above?

 

[ineedhelpnow]

(Blush) I am a little confused. i do understand what the graph of y=1/1+x^2 looks like.

 

[MarkFL]

(Blush) I am a little confused. i do understand what the graph of y=1/1+x^2 looks like.

Okay, now, do you think the shadow I described above would look like the graph of $$y=\frac{1}{1+x^2}$$ for the 3D graph you were given?

Thinking of shadows was the method I was taught back in the dark ages...:D