- #1
geft
- 148
- 0
Question: Evaluate using Green's Theorem and sketch R.
The question (excluding the sketch) and the attempted solution are on the attached image. I may have gotten the solution, but the numbers seem funny. Where did I go wrong?
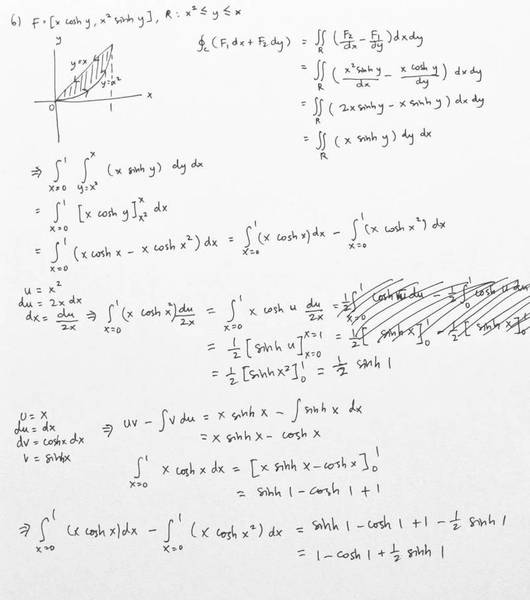
The question (excluding the sketch) and the attempted solution are on the attached image. I may have gotten the solution, but the numbers seem funny. Where did I go wrong?