Hamilton's principle and virtual work by constraint forces

[Kashmir]

Found a question on another website, I have the exact same question. Please help me

Goldstein says :

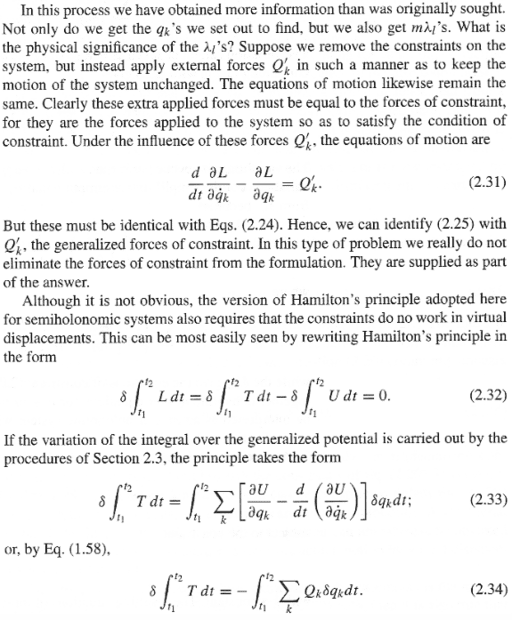
I do not understand how (2.34) shows that the virtual work done by forces of constraint is zero. How does the fact that "the same Hamilton's principle holds for both holonomic and semiholonomic systems" show that the additional forces of semiholonomic constraint do no work in the
$\delta q_k$

---

 

[andresB]

Contraints that do zero net virtual work are sometimes called ideal constraints. Not all constraints are ideal (https://hal.archives-ouvertes.fr/hal-01399622/document)

Here Goldstein is asuming ideal constraints (the work of the forces of constraint do not appear in the right hand side of 2.34).

 

[wrobel]

aints that do zero net virtual work are sometimes called ideal constraints. Not all constraints are ideal (https://hal.archives-ouvertes.fr/hal-01399622/document)

these guys completely do not understand what the D'Alembert-Lagrange is.
They think that they invented "nonideal constraints" but actually they consider systems with ideal constraints and given active forces applied. Some people begin to write articles before reading textbooks :)

 

[Kashmir]

these guys completely do not understand what the D'Alembert-Lagrange is.
They think that they invented "nonideal constraints" but actually they consider systems with ideal constraints and given active forces applied. Some people begin to write articles before reading textbooks :)

So what does the author mean? I still didn't get it