gmaverick2k
- 42
- 3
Summary:: Heat capacity for real gas with ideal gas (zero pressure) equation
I'm looking at this problem and I'm stuck.
I usually question everything but this problem is confusing me.
I don't know how they've made the jump from reduced properties (from generalized Cp charts(?)) to Cp-Cp°=1.70 cal/molK
Usually Cp-Cv=R
I've searched online and it seems Cp-Cv=ZR
This is not the same as Cp-Cp°=ZR, which would mean they've made Cp°=Cv (zero pressure so this is true?)
Has the author got the compressibility factor from the generalised compressibility chart for the Pr and Tr (Z=0.92)
I'm confused how they've made something straight forward
Below is the problem plus the authors solution:
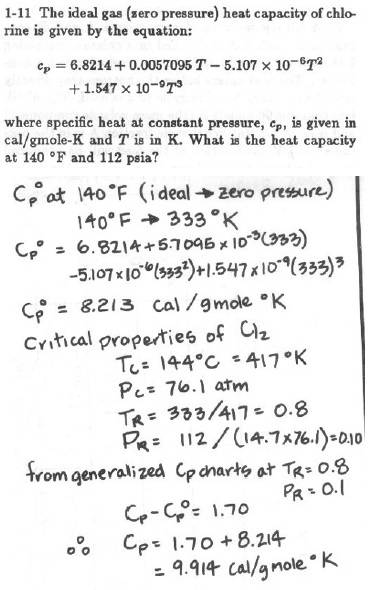
I'm looking at this problem and I'm stuck.
I usually question everything but this problem is confusing me.
I don't know how they've made the jump from reduced properties (from generalized Cp charts(?)) to Cp-Cp°=1.70 cal/molK
Usually Cp-Cv=R
I've searched online and it seems Cp-Cv=ZR
This is not the same as Cp-Cp°=ZR, which would mean they've made Cp°=Cv (zero pressure so this is true?)
Has the author got the compressibility factor from the generalised compressibility chart for the Pr and Tr (Z=0.92)
I'm confused how they've made something straight forward
Below is the problem plus the authors solution: