Alexey_H
- 8
- 1
- TL;DR Summary
- Is it possible to calculate heat loss improvements by knowing how the surface temperature changed?
Hello,
My house has some heat loss. As an example I know the outside door temperature. How can I calculate the heat loss delta if I reduce the door temperature by 1 degree?
I know that there is a formula to calculate a heat loss based on the U-value of a fabric. But I can't get those values as the walls and even doors are not made from a solid material. So in most cases it is not possible for me to get U-values.
So I thought if it is possible to calculate it based on temperature of surfaces?
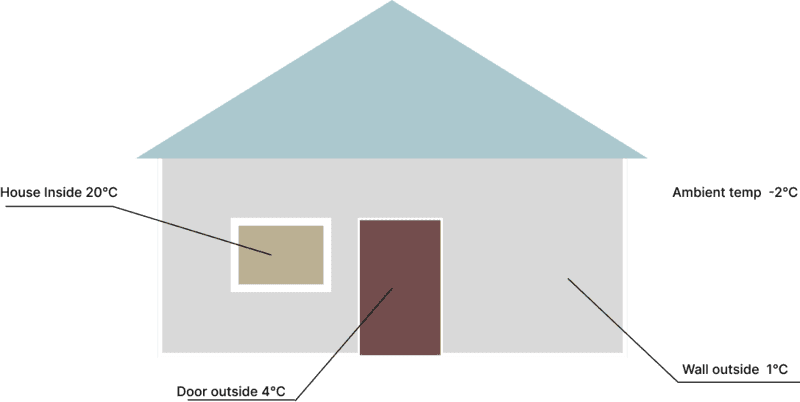
Details:
* Ambient temp: -2°C
* Door outside temp 4°C
* Door inside temp 20°C
* Door height: 2.2m
* Door width: 0.8m
How many watts could be saved by decreasing heat loss if I insulate the door and its outside temperature drops to 3°C ?
I need this to reason the house insulation in many places to my landlord. As now he is not sure if it makes sense but I pay a lot on my electricity bills.
I would appreciate any help with this.
My house has some heat loss. As an example I know the outside door temperature. How can I calculate the heat loss delta if I reduce the door temperature by 1 degree?
I know that there is a formula to calculate a heat loss based on the U-value of a fabric. But I can't get those values as the walls and even doors are not made from a solid material. So in most cases it is not possible for me to get U-values.
So I thought if it is possible to calculate it based on temperature of surfaces?
Details:
* Ambient temp: -2°C
* Door outside temp 4°C
* Door inside temp 20°C
* Door height: 2.2m
* Door width: 0.8m
How many watts could be saved by decreasing heat loss if I insulate the door and its outside temperature drops to 3°C ?
I need this to reason the house insulation in many places to my landlord. As now he is not sure if it makes sense but I pay a lot on my electricity bills.
I would appreciate any help with this.
