Heat, Work, and Energy: Understanding Charles Law and Internal Energy

[godingly]

1) I don't completely understands Charles Law (V = T*k) - This implies that if we increase the volume of an object, it's temperature will rise! Imagine a piston-apparatus - If we push the piston up, and increase the volume of the gas, why would its average kinetic energy rise? What would make the particles (on average) move faster?

2) I've read an example about heat, work and energy. It shows you can change a gas internal energy by either work or heat.

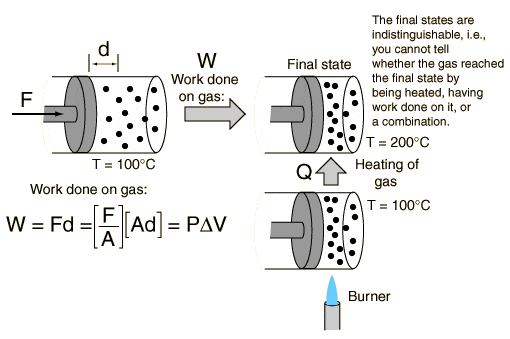
I don't understand why either of these changes will cause a rise in temperature:
a) In the work case - the gas's volume decreases, so therefore the gas's temperature should decrease as well, not increase! What's happening?
b) In the burner case - I don't understand what happens on the atomic level. I would appreciate an atomic-view explanation of what happens from when you press the burner button to the rise of the gas atoms average kinetic energy.

Thank you,
docendo discimus

 

[dauto]

Charles' law assumes constant pressure. If you increase the temperature the gas expands under constant pressure. The examples you provided do not have constant pressure so they are not going to follow Charles' law

 

[Andrew Mason]

I don't understand why either of these changes will cause a rise in temperature:
a) In the work case - the gas's volume decreases, so therefore the gas's temperature should decrease as well, not increase! What's happening?

The first law of thermodynamics is happening. Q = ΔU - W where W is the work done ON the gas. If Q = 0 (no heat flow into or out of the gas) then ΔU = W. So if there is work done ON the gas, internal energy, U, increases.

b) In the burner case - I don't understand what happens on the atomic level. I would appreciate an atomic-view explanation of what happens from when you press the burner button to the rise of the gas atoms average kinetic energy.

It is all about transfer of kinetic energy by molecular collisions.

The very fast moving molecules in the hot gases in the flame collide with the molecules in the container wall. This gets the molecules in the container moving very fast. The molecules in the container give up some of that kinetic energy to the contents, ie. the gas.

AM

 

[godingly]

Thank you, I understand the formula of the 1st law, but I don't understand :
1) In the case of work, the ideal gas law states PV=nRt. since n & R are constant, and T rises, that means Pressure increases by more than Volume decreases. But Boyle's law states that V and P change inversely proportionally - how is this possible?
2) How, in the microscopic level, would the work increase the average kinetic energy of the atoms? Is it because we increased the pressure on the piston, and now the piston atoms move faster, and then when the gas atoms heat the piston atoms - the gas atoms would move faster?

 

[dauto]

1) Boyle's law assumes the temperature is being kept constant. That's not the case here as you pointed out so Boyle's law doesn't apply. When learning laws such as Crarles' law and Boyle's law you ought to also learn the assumptions that go into the laws so you know when to apply the laws and when not to apply them

2) Yes, that's correct.

 

[Andrew Mason]

2) How, in the microscopic level, would the work increase the average kinetic energy of the atoms? Is it because we increased the pressure on the piston, and now the piston atoms move faster, and then when the gas atoms heat the piston atoms - the gas atoms would move faster?

Just as a moving bat causes a baseball to rebound with more energy than a stationary bat, the moving container wall increases the rebound energy of the molecules that collide with it.

The difference is that with a gas there are many molecules. If the wall is moving slowly, the moving wall increases the speed of each molecule less, but many more molecules are affected because the wall is moving for a longer time.

AM