Help understanding rc circuits?

[jaredvert]

Help understanding rc circuits?

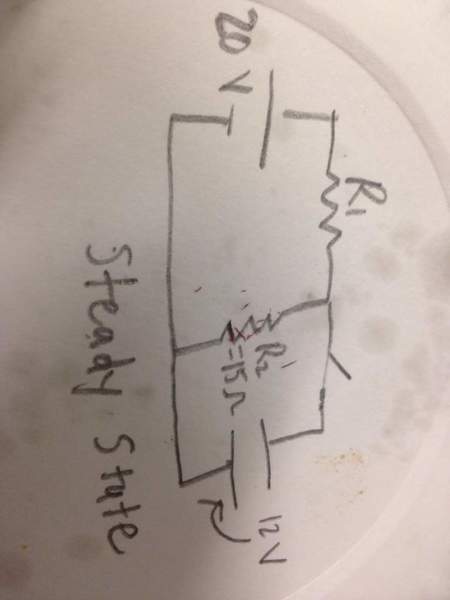so I know the voltage drop must be 8 v through the r1 resistor because of the 12 v charge on the capacitor. 8= r1*i and now I set up another equation 12= r2*i= 15*i and when I solve for the current I get 4/5 a but my textbook lists 3 amps as correct. Can you help me here please? Please explain all concepts as well because I might be a little shaky. Should I apply Kirchhoff's rule? Thanks

 

[phinds]

If R2 is indeed 15 ohms, there is no possibility that it has 3 amps flowing through it in that circuit. Are you sure it wasn't supposed to be 1.5 ohms? Come to think of it, even then the answer can't be 3 amps. Are you sure you've stated the problem correctly?

 

[jaredvert]

Wait is 4/5 ohms right for this set up?

 

[jaredvert]

If R2 is indeed 15 ohms, there is no possibility that it has 3 amps flowing through it in that circuit. Are you sure it wasn't supposed to be 1.5 ohms? Come to think of it, even then the answer can't be 3 amps. Are you sure you've stated the problem correctly?

So is 4/5 ohms right for this set up?

 

[UltrafastPED]

Wait is 4/5 ohms right for this set up?

Well, 4/5 amp; yes our analyses agree.

If you label the node below R2 as (Ground), and the one above R2 as (A), and close the switch:

1. Then node (A) is at 12 volts
2. There is a 12 volt drop across resistor R2, so the current is 12 volts/15 ohms = 4/5 amp.
3. There is an 8 volt drop across resistor R1, so R1 = 2/3 R2 = 10 ohms.

Check: current in the loop is 20 volts/(10+15) ohms = 4/5 amp

Have your teacher check the textbook errata; or perhaps you have done the wrong problem!

 

[jaredvert]

Well, 4/5 amp; yes our analyses agree.

Ok well then idk what's going on. My textbook has this same set up and says 3 amps. Anyways did my analysis match up with yours about the 8 v and 12 because I think I'm getting the hang of it but am I correct in saying at steady state this set up turns into a series because no current then goes through that capacitor. Now what if another resistor was next to the capacitor in the same branch. Would there still be a current in that "stem"/branch and would there be less q/v on the capacitor because of the pot drop? Thanks

 

[Drakkith]

Ok well then idk what's going on. My textbook has this same set up and says 3 amps. Anyways did my analysis match up with yours about the 8 v and 12 because I think I'm getting the hang of it but am I correct in saying at steady state this set up turns into a series because no current then goes through that capacitor.

Correct. No current flows through the capacitor once it reaches steady state.

Now what if another resistor was next to the capacitor in the same branch. Would there still be a current in that "stem"/branch and would there be less q/v on the capacitor because of the pot drop? Thanks

If the resistor is in parallel with both the capacitor and R2 then yes, there is current flow through the resistor. However, adding the resistor lowers the equivalent resistance of the whole parallel branch, meaning that each component has less than 12 volts of voltage drop. The voltage drop across R1 increases since it makes up a greater proportion of the circuits resistance now.

For example, adding another 15 ohm resistor (call it R3) means that the equivalent resistance of the parallel branch is actually only 7.5 ohms. Since R1 is 10 ohms, that means that total equivalent resistance of the circuit is now 17.5 ohms. So current through the circuit is 20v/17.5 ohms = 1.14 amps.

Rearranging ohms law, we see that R1 now has 11.4 volts across it (1.14 amps x 10 ohms), leaving 8.6 volts across R2, R3, and C1. 8.6 volts across R2 and R3 gives 0.57 amps of current through each (8.6v/15 ohms = 0.57 amps), which adds up to the 1.14 amps of total current through the circuit.

C1, being in parallel with R2 and R3, would only have 8.6 volts across it and would have less charge on each plate than before.

 

[jaredvert]

Correct. No current flows through the capacitor once it reaches steady state.

If the resistor is in parallel with both the capacitor and R2 then yes, there is current flow through the resistor. However, adding the resistor lowers the equivalent resistance of the whole parallel branch, meaning that each component has less than 12 volts of voltage drop. The voltage drop across R1 increases since it makes up a greater proportion of the circuits resistance now.
For example, adding another 15 ohm resistor (call it R3) means that the equivalent resistance of the parallel branch is actually only 7.5 ohms. Since R1 is 10 ohms, that means that total equivalent resistance of the circuit is now 17.5 ohms. So current through the circuit is 20v/17.5 ohms = 1.14 amps.
Rearranging ohms law, we see that R1 now has 11.4 volts across it (1.14 amps x 10 ohms), leaving 8.6 volts across R2, R3, and C1. 8.6 volts across R2 and R3 gives 0.57 amps of current through each (8.6v/15 ohms = 0.57 amps), which adds up to the 1.14 amps of total current through the circuit.
C1, being in parallel with R2 and R3, would only have 8.6 volts across it and would have less charge on each plate than before.

I meant if you put a new resistor right under the capacitor on the same branch. Would that make a voltage drop across that resistor and make less pot diff across that same capacitor?

 

[Drakkith]

I meant if you put a new resistor right under the capacitor on the same branch. Would that make a voltage drop across that resistor and make less pot diff across that same capacitor?

What do you mean "right under" the capacitor? Is it in series with the capacitor?